A Treatise on Electricity and Magnetism/Volume 1/Part 1/Chapter 11

by James Clerk Maxwell

CHAPTER XI.

THEORY OF ELECTRIC IMAGES AND ELECTRIC INVERSION.

155.] We have already shown that when a conducting sphere is under the influence of a known distribution of electricity, the distribution of electricity on the surface of the sphere can be determined by the method of spherical harmonics.

For this purpose we require to expand the potential of the influencing system in a series of solid harmonics of positive degree, having as the centre of the sphere as origin and we then find a corresponding series of solid harmonics of negative degree, which express the potential due to the electrification of the sphere.

By the use of this powerful method of analysis, Poisson determined the electrification of a sphere under the influence of a given electrical system and he also solved the more difficult problem to determine the distribution of electricity on two conducting spheres in presence of each other. These investigations have been pursued at great length by Plana and others, who have confirmed the accuracy of Poisson.

In applying this method to the most elementary case of a sphere under the influence of a single electrified point, we require to expand the potential due to the electrified point in a series of solid harmonics, and to determine a second series of solid harmonics which express the potential, due to the electrification of the sphere, in the space outside.

It does not appear that any of these mathematicians observed that this second series expresses the potential due to an imaginary electrified point, which as no physical existence as an electrified point, but which may be called an electrical image, because the action of the surface on external points is the same as that which would be produced by the imaginary electrified point if the spherical surface were removed.

This discovery seems to have been reserved for Sir W. Thomson, who has developed it into a method of great power for the solution of electrical problems, and at the same time capable of being presented in an elementary geometrical form.

His original investigations, which are contained in the Cambridge and Dublin Mathematical Journal, 1848, are expressed in terms of the ordinary theory of attraction at a distance, and make no use of the method of potentials and of the general theorems of Chapter IV, though they were probably discovered by these methods. Instead, however, of following the method of the author, I shall make free use of the idea of the potential and of equipotential surfaces, when ever the investigation can be rendered more intelligible by such means.

Theory of Electric Images.

Fig. 7

156.] Let $A$ and $B$, Figure 7, represent two points in a uniform dielectric medium of infinite extent. Let the charges of $A$ and $B$ be $e_{1}$ and $e_{2}$ respectively. Let $P$ be any point in space whose distances from $A$ and $B$ are $r_{1}$ and $r_{2}$ respectively. Then the value of the potential at $P$ will be

$V={\frac {e_{1}}{r_{1}}}+{\frac {e_{2}}{r_{2}}}.$

The equipotential surfaces due to this distribution of electricity are represented in Fig. I (at the end of this volume) when $e_{1}$ and $e_{2}$ are of the same sign, and in Fig. II when they are of opposite signs. We have now to consider that surface for which $V=0$, which is the only spherical surface in the system. When $e_{1}$ and $e_{2}$ are of the same sign, this surface is entirely at an infinite distance, but when they are of opposite signs there is a plane or spherical surface at a finite distance for which the potential is zero.

Fig. I

||

Fig. II

The equation of this surface is

${\frac {e_{1}}{r_{1}}}+{\frac {e_{2}}{r_{2}}}=0$

(2)

Its centre is at a point C in AB produced, such that

$AC:BC::e_{1}^{2}:e_{2}^{2},$

and the radius of the sphere is

$AB{\frac {e_{1}e_{2}}{e_{1}^{2}-e_{2}^{2}}}.$

The two points A and B are inverse points with respect to this sphere, that is to say, they lie in the same radius, and the radius is a mean proportional between their distances from the centre.

Since this spherical surface is at potential zero, if we suppose it constructed of thin metal and connected with the earth, there will be no alteration of the potential at any point either outside or inside, but the electrical action everywhere will remain that due to the two electrified points $A$ and $B$.

If we now keep the metallic shell in connexion with the earth and remove the point $B$, the potential within the sphere will become everywhere zero, but outside it will remain the same as before. For the surface of the sphere still remains at the same potential, and no change has been made in the exterior electrification.

Hence, if an electrified point $A$ be placed outside a spherical conductor which is at potential zero, the electrical action at all points outside the sphere will be that due to the point $A$ together with another point $B$ within the sphere, which we may call the electrical image of $A$.

In the same way we may shew that if $B$ is a point placed inside the spherical shell, the electrical action within the sphere is that due to $B$, together with its image $A$.

157.] Definition of an Electrical Image. An electrical image is an electrified point or system of points on one side of a surface which would produce on the other side of that surface the same electrical action which the actual electrification of that surface really does produce.

In Optics a point or system of points on one side of a mirror or lens which if it existed would emit the system of rays which actually exists on the other side of the mirror or lens, is called a virtual image.

Electrical images correspond to virtual images in optics in being related to the space on the other side of the surface. They do not correspond to them in actual position, or in the merely approximate character of optical foci.

There are no real electrical images, that is, imaginary electrified points which would produce, in the region on the same side of the electrified surface, an effect equivalent to that of the electrified surface.

For if the potential in any region of space is equal to that due to a certain electrification in the same region it must be actually produced by that electrification. In fact, the electrification at any point may be found from the potential near that point by the application of Poisson's equation.

Let $a$ be the radius of the sphere.

Let $f$ be the distance of the electrified point $A$ from the centre $C$.

Let $e$ be the charge of this point.

Then the image of the point is at $B$, on the same radius of the sphere at a distance ${\tfrac {a^{2}}{f}}$, and the charge of the image is $-e{\tfrac {a}{f}}$.

Fig. 7

We have shewn that this image will produce the same effect on the opposite side of the surface as the actual electrification of the surface does. We shall next determine the surface-density of this electrification at any point $P$ of the spherical surface, and for this purpose we shall make use of the theorem of Coulomb, Art. 80, that if $R$ is the resultant force at the surface of a conductor, and $\sigma$ the superficial density,

$R=4\pi \sigma ,\,$

$R$ being measured away from the surface.

We may consider $R$ as the resultant of two forces, a repulsion ${\tfrac {e}{AP^{2}}}$ acting along $AP$, and an attraction $e{\frac {a}{f}}{\frac {1}{PB^{2}}}$ acting along $PB$.

Resolving these forces in the directions of $AC$ and $CP$, we find that the components of the repulsion are

${\frac {ef}{AP^{3}}}$ along AC, and ${\frac {ea}{AP^{3}}}$ along CP.

Those of the attraction are

$-e{\frac {a}{f}}{\frac {1}{BP^{3}}}$ along AC, and $-e{\frac {a^{2}}{f}}{\frac {1}{BP^{3}}}$ along CP.

Now $BP={\tfrac {a}{f}}AP$, and $BC={\tfrac {a^{2}}{f}}$, so that the components of the attraction may be written

$-ef{\frac {1}{AP^{3}}}$ along AC, and $-e{\frac {f^{2}}{a}}{\frac {1}{AP^{3}}}$ along CP.

The components of the attraction and the repulsion in the direction of $AC$ are equal and opposite, and therefore the resultant force is entirely in the direction of the radius $CP$. This only confirms what we have already proved, that the sphere is an equipotential surface, and therefore a surface to which the resultant force is everywhere perpendicular.

The resultant force measured along $CP$, the normal to the surface in the direction towards the side on which $A$ is placed, is

$R=-e{\frac {f^{2}-a^{2}}{a}}{\frac {1}{AP^{3}}}$

(3)

If $A$ is taken inside the sphere $f$ is less than $a$, and we must measure $R$ inwards. For this case therefore

$R=-e{\frac {a^{2}-f^{2}}{a}}{\frac {1}{AP^{3}}}$

(4)

In all cases we may write

$R=-e{\frac {AD\cdot Ad}{CP}}{\frac {1}{AP^{3}}},$

(5)

where $AD,Ad$ are the segments of any line through $A$ cutting the sphere, and their product is to be taken positive in all cases.

158.] From this it follows, by Coulomb's theorem, Art. 80, that the surface-density at $P$ is

$\sigma =-e{\frac {AD\cdot Ad}{4\pi \cdot CP}}{\frac {1}{AP^{3}}}$

(6)

The density of the electricity at any point of the sphere varies inversely as the cube of its distance from the point $A$.

The effect of this superficial distribution, together with that of the point $A$, is to produce on the same side of the surface as the point $A$ a potential equivalent to that due to $e$ at $A$, and its image $-e{\tfrac {a}{f}}$ at $B$, and on the other side of the surface the potential is everywhere zero. Hence the effect of the superficial distribution by itself is to produce a potential on the side of $A$ equivalent to that due to the image $-e{\tfrac {a}{f}}$ at $B$, and on the opposite side a potential equal and opposite to that of $e$ at $A$.

The whole charge on the surface of the sphere is evidently $-e{\tfrac {a}{f}}$ since it is equivalent to the image at $B$.

We have therefore arrived at the following theorems on the action of a distribution of electricity on a spherical surface, the surface-density being inversely as the cube of the distance from a point $A$ either without or within the sphere.

Let the density be given by the equation

$\sigma ={\frac {C}{AP^{3}}}$

(7)

where $C$ is some constant quantity, then by equation (6)

$C=-e{\frac {AD\cdot Ad}{4\pi a}}$

(8)

The action of this superficial distribution on any point separated from $A$ by the surface is equal to that of a quantity of electricity $-e$, or

${\frac {4\pi aC}{AD\cdot Ad}}$

concentrated at $A$.

Its action on any point on the same side of the surface with $A$ is equal to that of a quantity of electricity

${\frac {4\pi Ca^{2}}{f\ AD\cdot Ad}}$

concentrated at $B$ the image of $A$.

The whole quantity of electricity on the sphere is equal to the first of these quantities if $A$ is within the sphere, and to the second if $A$ is without the sphere.

These propositions were established by Sir W. Thomson in his original geometrical investigations with reference to the distribution of electricity on spherical conductors, to which the student ought to refer.

159.] If a system in which the distribution of electricity is known is placed in the neighbourhood of a conducting sphere of radius $a$, which is maintained at potential zero by connexion with the earth, then the electrifications due to the several parts of the system will be superposed.

Let $A_{1},A_{2}$, &c. be the electrified points of the system, $f_{1},f_{2}$ &c. their distances from the centre of the sphere, $e_{1},e_{2}$, &c. their charges, then the images $B_{1},B_{2}$, &c. of these points will be in the same radii as the points themselves, and at distances ${\tfrac {a^{2}}{f_{1}}},\ {\tfrac {a^{2}}{f_{2}}}$ &c. from the centre of the sphere, and their charges will be

$-e{\frac {a}{f_{1}}},\ -e{\frac {a}{f_{2}}}\ etc.$

The potential on the outside of the sphere due to the superficial electrification will be the same as that which would be produced by the system of images $B_{1},B_{2}$, &c. This system is therefore called the electrical image of the system $A_{1},A_{2}$, &c.

If the sphere instead of being at potential zero is at potential $V$, we must superpose a distribution of electricity on its outer surface having the uniform surface-density

$\sigma ={\frac {V}{4\pi a}}$

The effect of this at all points outside the sphere will be equal to that of a quantity $Va$ of electricity placed at its centre, and at all points inside the sphere the potential will be simply increased by $V$.

The whole charge on the sphere due to an external system of influencing points $A_{1},A_{2}$, &c. is

$E=Va-e_{1}{\frac {a}{f_{1}}}-e_{2}{\frac {a}{f_{2}}}-etc.,$

(9)

from which either the charge $E$ or the potential $V$ may be calculated when the other is given.

When the electrified system is within the spherical surface the induced charge on the surface is equal and of opposite sign to the inducing charge, as we have before proved it to be for every closed surface, with respect to points within it.

160.] The energy due to the mutual action between an electrified point $e$, at a distance $f$ from the centre of the sphere greater than $a$ the radius, and the electrification of the spherical surface due to the influence of the electrified point and the charge of the sphere, is

$M=e\left({\frac {Va}{f}}-{\frac {ea}{f^{2}-a^{2}}}\right)={\frac {e}{f}}\left(E-{\frac {e^{2}a^{3}}{f\left(f^{2}-a^{2}\right)}}\right),$

(10)

where $V$ is the potential, and $E$ the charge of the sphere.

The repulsion between the electrified point and the sphere is therefore, by Art. 92,

${\begin{array}{ll}F&=ea\left({\frac {V}{f^{2}}}-{\frac {ef}{\left(f^{2}-a^{2}\right)^{2}}}\right)\\\\&={\frac {e}{f^{2}}}\left(E-e{\frac {a^{3}\left(2f^{2}-a^{2}\right)}{f\left(f^{2}-a^{2}\right)^{2}}}\right).\end{array}}$

(11)

Hence the force between the point and the sphere is always an attraction in the following cases–

(1) When the sphere is uninsulated.

(2) When the sphere has no charge.

(3) When the electrified point is very near the surface.

In order that the force may be repulsive, the potential of the sphere must be positive and greater than $e{\tfrac {f^{3}}{\left(f^{2}-a^{2}\right)^{2}}}$, and the charge of the sphere must be of the same sign as $e$ and greater than $e{\tfrac {a^{3}\left(2f^{2}-a^{2}\right)}{f\left(f^{2}-a^{2}\right)^{2}}}$.

At the point of equilibrium the equilibrium is unstable, the force being an attraction when the bodies are nearer and a repulsion when they are farther off.

When the electrified point is within the spherical surface the force on the electrified point is always away from the centre of the sphere, and is equal to

${\frac {e^{2}af}{\left(a^{2}-f^{2}\right)^{2}}}.$

The surface-density at the point of the sphere nearest to the electrified point where it lies outside the sphere is

${\begin{array}{ll}\sigma _{1}&={\frac {1}{4\pi a^{2}}}\left\{Va-e{\frac {a(f+a)}{(f-a)^{2}}}\right\}\\\\&={\frac {1}{4\pi a^{2}}}\left\{E-e{\frac {a^{2}(3f-a)}{f(f-a)^{2}}}\right\}.\end{array}}$

(12)

The surface-density at the point of the sphere farthest from the electrified point is

${\begin{array}{ll}\sigma _{2}&={\frac {1}{4\pi a^{2}}}\left\{Va-e{\frac {a(f-a)}{(f+a)^{2}}}\right\}\\\\&={\frac {1}{4\pi a^{2}}}\left\{E+e{\frac {a^{2}(3f+a)}{f(f+a)^{2}}}\right\}.\end{array}}$

(13)

When $E$, the charge of the sphere, lies between

$e{\frac {a^{2}(3f-a)}{f(f-a)^{2}}}$ and $-e{\frac {a^{2}(3f+a)}{f(f+a)^{2}}}$

the electrification will be negative next the electrified point and positive on the opposite side. There will be a circular line of division between the positively and the negatively electrified parts of the surface, and this line will be a line of equilibrium.

If

$E=ea\left({\frac {1}{\sqrt {f^{2}-a^{2}}}}-{\frac {1}{f}}\right),$

(14)

the equipotential surface which cuts the sphere in the line of equilibrium is a sphere whose centre is the electrified point and whose radius is ${\sqrt {f^{2}-a^{2}}}$.

The lines of force and equipotential surfaces belonging to a case of this kind are given in Figure IV at the end of this volume.

Images in an Infinite Plane Conducting Surface.

161.] If the two electrified points $A$ and $B$ in Art. 156 are electrified with equal charges of electricity of opposite signs, the surface of zero potential will be the plane, every point of which is equidistant from $A$ and $B$.

Fig. 8

Hence, if $A$ be an electrified point whose charge is $e$, and $AD$ a perpendicular on the plane, produce $AD$ to $B$ so that $DB=AB$, and place at $B$ a charge equal to $-e$, then this charge at $B$ will be the image of $A$, and will produce at all points on the same side of the plane as $A$, an effect equal to that of the actual electrification of the plane. For the potential on the side of $A$ due to $A$ and $B$ fulfils the conditions that $\nabla ^{2}V=0$ everywhere except at $A$, and that $V=0$ at the plane, and there is only one form of $V$ which can fulfil these conditions.

To determine the resultant force at the point $P$ of the plane, we observe that it is compounded of two forces each equal to ${\tfrac {e}{AP^{2}}}$, one acting along $AP$ and the other along $PB$. Hence the resultant of these forces is in a direction parallel to $AB$ and equal to

${\frac {e}{AP^{2}}}\cdot {\frac {AB}{AP}}$

Hence $R$, the resultant force measured from the surface towards the space in which $A$ lies, is

$R=-{\frac {2eAD}{AP^{3}}}$

(15)

and the density at the point $P$ is

$\sigma =-{\frac {eAD}{2\pi AP^{3}}}.$

(16)

On Electrical Inversion.

162.] The method of electrical images leads directly to a method of transformation by which we may derive from any electrical problem of which we know the solution any number of other problems with their solutions.

We have seen that the image of a point at a distance $r$ from the centre of a sphere of radius $R$ is in the same radius and at a distance $r'$ such that $rr'=R^{2}$. Hence the image of a system of points, lines, or surfaces is obtained from the original system by the method known in pure geometry as the method of inversion, and described by Chasles, Salmon, and other mathematicians.

Fig. 9

If $A$ and $B$ are two points, $A'$ and $B'$ their images, $O$ being the centre of inversion, and $R$ the radius of the sphere of inversion,

$OA\cdot OA'=R^{2}=OB\cdot OB'$

Hence the triangles $OAB$, $OB'A'$ are similar, and

$AB:A'B'::OA:OB'::OA\cdot OB:R^{2}.$

If a quantity of electricity $e$ be placed at $A$, its potential at $B$ will be

$V={\frac {e}{AB}}$

If $e'$ be placed at $A'$ its potential at $B'$ will be

$V'={\frac {e'}{A'B'}}.$

In the theory of electrical images

$e:e'::OA:R::R:OA'.$

Hence

$V:V'::R:OB,\,$

(17),

or the potential at $B$ due to the electricity at $A$ is to the potential at the image of $B$ due to the electrical image of $A$ as $R$ is to $OB$.

Since this ratio depends only on $OB$ and not on $OA$, the potential at $B$ due to any system of electrified bodies is to that at $B'$ due to the image of the system as $R$ is to $OB$.

If $r$ be the distance of any point $A$ from the centre, and $r'$ that of its image $A'$, and if $e$ be the electrification of $A$, and $e'$ that of $A'$, also if $L,S,K$ be linear, superficial, and solid elements at $A$, and $L',S',K'$ their images at $A'$, and $\lambda ,\sigma ,\rho ,\lambda ',\sigma ',\rho ',$ the corresponding line-surface and volume-densities of electricity at the two points, $V$ the potential at $A$ due to the original system, and $V'$ the potential at $A'$ due to the inverse system, then

$\left.{\begin{array}{c}{\frac {r'}{r}}={\frac {L'}{L}}={\frac {R^{2}}{r^{2}}}={\frac {r'^{2}}{r^{2}}},\ {\frac {S'}{S}}={\frac {R^{4}}{r^{4}}}={\frac {r'^{4}}{R^{4}}},\ {\frac {K'}{K}}={\frac {R^{6}}{r^{6}}}={\frac {r'^{6}}{R^{6}}};\\\\{\frac {e'}{e}}={\frac {R}{r}}={\frac {r'}{R}},\ {\frac {\lambda '}{\lambda }}={\frac {r}{R}}={\frac {R}{r'}},\\\\{\frac {\sigma '}{\sigma }}={\frac {r^{3}}{R^{3}}}={\frac {R^{3}}{r'^{3}}},\ {\frac {\rho '}{\rho }}={\frac {r^{5}}{R^{5}}}={\frac {R^{5}}{r'^{5}}}\\\\{\frac {V'}{V}}={\frac {r}{R}}={\frac {R}{r'}}.\end{array}}\right\}$

(18)[1]

If in the original system a certain surface is that of a conductor, and has therefore a constant potential $P$, then in the transformed system the image of the surface will have a potential $P{\tfrac {R}{r'}}$. But by placing at $O$, the centre of inversion, a quantity of electricity equal to $-PR$, the potential of the transformed surface is reduced to zero.

Hence, if we know the distribution of electricity on a conductor when insulated in open space and charged to the potential $P$, we can find by inversion the distribution on a conductor whose form is the image of the first under the influence of an electrified point with a charge $-PR$ placed at the centre of inversion, the conductor being in connexion with the earth.

163.] The following geometrical theorems are useful in studying cases of inversion.

Every sphere becomes, when inverted, another sphere, unless it passes through the centre of inversion, in which case it becomes a plane.

If the distances of the centres of the spheres from the centre of inversion are $a$ and $a'$, and if their radii are $\alpha$ and $\alpha '$, and if we define the power of the sphere with respect to the centre of in version to be the product of the segments cut off by the sphere from a line through the centre of inversion, then the power of the first sphere is $a^{2}-\alpha ^{2}$, and that of the second is $a'^{2}-\alpha '^{2}$. We have in this case

${\frac {a'}{a}}={\frac {\alpha '}{\alpha }}={\frac {R^{2}}{a^{2}-\alpha ^{2}}}={\frac {a'^{2}-\alpha '^{2}}{R^{2}}},$

(19)

or the ratio of the distances of the centres of the first and second spheres is equal to the ratio of their radii, and to the ratio of the power of the sphere of inversion to the power of the first sphere, or of the power of the second sphere to the power of the sphere of inversion.

The centre of either sphere corresponds to the inverse point of the other with respect to the centre of inversion.

In the case in which the inverse surfaces are a plane and a sphere, the perpendicular from the centre of inversion on the plane is to the radius of inversion as this radius is to the diameter of the sphere, and the sphere has its centre on this perpendicular and passes through the centre of inversion.

Every circle is inverted into another circle unless it passes through the centre of inversion, in which case it becomes a straight line.

The angle between two surfaces, or two lines at their intersection, is not changed by inversion.

Every circle which passes through a point, and the image of that point with respect to a sphere, cuts the sphere at right angles.

Hence, any circle which passes through a point and cuts the sphere at right angles passes through the image of the point.

164.] We may apply the method of inversion to deduce the distribution of electricity on an uninsulated sphere under the influence of an electrified point from the uniform distribution on an insulated sphere not influenced by any other body.

If the electrified point be at $A$, take it for the centre of inversion, and if $A$ is at a distance $f$ from the centre of the sphere whose radius is $a$, the inverted figure will be a sphere whose radius is $a'$ and whose centre is distant $f'$, where

${\frac {a'}{a}}={\frac {f'}{f}}={\frac {R^{2}}{f^{2}-a^{2}}}.$

(20)

The centre of either of these spheres corresponds to the inverse point of the other with respect to $A$, or if $C$ is the centre and $B$ the inverse point of the first sphere, $C'$ will be the inverse point, and $B'$ the centre of the second.

Now let a quantity $e'$ of electricity be communicated to the second sphere, and let it be uninfluenced by external forces. It will become uniformly distributed over the sphere with a surface-density

$\sigma '={\frac {e'}{4\pi a'^{2}}}.$

(21)

Its action at any point outside the sphere will be the same as that of a charge $e'$ placed at $B'$ the centre of the sphere.

At the spherical surface and within it the potential is

$P'={\frac {e'}{a'}},$

(22)

a constant quantity.

Now let us invert this system. The centre $B'$ becomes in the inverted system the inverse point $B$, and the charge $e'$ at $B'$ becomes $e'{\tfrac {AB}{R}}$ at $B$, and at any point separated from $B$ by the surface the potential is that due to this charge at $B$.

The potential at any point $P$ on the spherical surface, or on the same side as $B$, is in the inverted system

${\frac {e'}{a'}}{\frac {R}{AP}}$

If we now superpose on this system a charge $e$ at $A$, where

$e=-{\frac {e'}{a'}}R,$

(23)

the potential on the spherical surface, and at all points on the same side as $B$, will be reduced to zero. At all points on the same side as $A$ the potential will be that due to a charge $e$ at $A$, and a charge $e'{\frac {R}{f'}}$ at $B$.

But

$e'{\frac {R}{f'}}=-e{\frac {a'}{f'}}=-e{\frac {a}{f}},$

(24)

as we found before for the charge of the image at $B$.

To find the density at any point of the first sphere we have

$\sigma =\sigma '{\frac {R^{3}}{AP^{3}}}.$

(25)

Substituting for the value of $\sigma '$ in terms of the quantities be longing to the first sphere, we find the same value as in Art. 158,

$\sigma =-{\frac {e\left(f^{2}-a^{2}\right)}{4\pi aAP^{3}}}.$

(26)

On Finite Systems of Successive Images.

165.] If two conducting planes intersect at an angle which is a submultiple of two right angles, there will be a finite system of images which will completely determine the electrification.

Fig. 10

For let $AOB$ be a section of the two conducting planes per pendicular to their line of inter section, and let the angle of intersection $AOB={\frac {\pi }{n}},$, let $P$ be an electrified point, and let $PO=r$, and $POB=\theta$. Then, if we draw a circle with centre and radius $OP$, and find points which are the successive images of $P$ in the two planes beginning with $OB$, we shall find $Q_{1}$ for the image of $P$ in $OB$, $P_{2}$ for the image of $Q_{1}$ in $OA$, $Q_{3}$ for that of $P_{2}$ in $OB$, $P_{3}$ for that of $Q_{3}$ in $OA$, and $Q_{2}$ for that of $P_{3}$ in $OB$.

If we had begun with the image of $P$ in $AO$ we should have found the same points in the reverse order $Q_{2},P_{3},Q_{3},P_{2},Q_{1}$, provided $AOB$ is a submultiple of two right angles.

For the alternate images $P_{1},P_{2},P_{3}$ are ranged round the circle at angular intervals equal to $2AOB$, and the intermediate images $Q_{1},Q_{2},Q_{3}$ are at intervals of the same magnitude. Hence, if $2AOB$ is a sub multiple of 2$\pi$, there will be a finite number of images, and none of these will fall within the angle $AOB$. If, however, $AOB$ is not a submultiple of it, it will be impossible to represent the actual electrification as the result of a finite series of electrified points.

If $AOB={\frac {\pi }{n}}$, there will be $n$ negative images $Q_{1},Q_{2},$ &c., each equal and of opposite sign to $P$, and $n-1$ positive images $P_{2},P_{3}$, &c., each equal to $P$, and of the same sign.

The angle between successive images of the same sign is ${\tfrac {2\pi }{n}}$. If we consider either of the conducting planes as a plane of symmetry, we shall find the positive and negative images placed symmetrically with regard to that plane, so that for every positive image there is a negative image in the same normal, and at an equal distance on the opposite side of the plane.

If we now invert this system with respect to any point, the two planes become two spheres, or a sphere and a plane intersecting at an angle ${\tfrac {\pi }{n}}$, the influencing point $P$ being within this angle.

The successive images lie on the circle which passes through $P$ and intersects both spheres at right angles.

To find the position of the images we may either make use of the principle that a point and its image are in the same radius of the sphere, and draw successive chords of the circle beginning at $P$ and passing through the centres of the two spheres alternately.

To find the charge which must be attributed to each image, take any point in the circle of intersection, then the charge of each image is proportional to its distance from this point, and its sign is positive or negative according as it belongs to the first or the second system.

166.] We have thus found the distribution of the images when any space bounded by a conductor consisting of two spherical surfaces meeting at an angle ${\tfrac {\pi }{n}}$, and kept at potential zero, is influenced by an electrified point.

We may by inversion deduce the case of a conductor consisting of two spherical segments meeting at a re-entering angle ${\tfrac {\pi }{n}}$, charged to potential unity and placed in free space.

For this purpose we invert the system with respect to $P$. The circle on which the images formerly lay now becomes a straight line through the centres of the spheres.

Fig. 11

If the figure (11) represents a section through the line of centres $AB$, and if $D,D'$ are the points where the circle of intersection cuts the plane of the paper, then, to find the successive images, draw $DA$ a radius of the first circle, and draw $DC,DB$, &c., making angles ${\frac {\pi }{n}},\ {\frac {2\pi }{n}}$, &c. with $DA$. The points $C,B$, &c. at which they cut the line of centres will he the positions of the positive images, and the charge of each will be represented by its distances from $D$. The last of these images will be at the centre of the second circle.

To find the negative images draw $DP,DQ$, &c., making angles ${\frac {\pi }{n}},\ {\frac {2\pi }{n}}$, &c. with the line of centres. The intersections of these lines with the line of centres will give the positions of the negative images, and the charge of each will be represented by its distance from $D$.

The surface-density at any point of either sphere is the sum of the surface-densities due to the system of images. For instance, the surface-density at any point $S$ of the sphere whose centre is $A$, is

$\sigma ={\frac {1}{4\pi DA}}\left\{1+\left(AD^{2}-AB^{2}\right){\frac {DB}{BS^{3}}}+\left(AD^{2}-AC^{2}\right){\frac {DC}{CS^{3}}}+etc.\right\},$

where $A,B,C$, &c. are the positive series of images.

When $S$ is on the circle of intersection the density is zero.

To find the total charge on each of the spherical segments, we may find the surface-integral of the induction through that segment due to each of the images.

The total charge on the segment whose centre is $A$ due to the image at $A$ whose charge is $DA$ is

$DA{\frac {DA+OA}{2DA}}={\frac {1}{2}}\left(DA+OA\right),$

where $O$ is the centre of the circle of intersection.

In the same way the charge on the same segment due to the image at $B$ is ${\tfrac {1}{2}}\left(DB+OB\right)$, and so on, lines such as $OB$ measured from to the left being reckoned negative.

Hence the total charge on the segment whose centre is A is

${\frac {1}{2}}(DA+DB+DC+etc.)+{\frac {1}{2}}(OA+OB+OC+etc.)$

$-{\frac {1}{2}}(DP+DQ+etc.)-{\frac {1}{2}}(OP+OQ+etc.)$

167.] The method of electrical images may be applied to any space bounded by plane or spherical surfaces all of which cut one another in angles which are submultiples of two right angles.

In order that such a system of spherical surfaces may exist, every solid angle of the figure must be trihedral, and two of its angles must be right angles, and the third either a right angle or a submultiple of two right angles.

Hence the cases in which the number of images is finite are–

(1) A single spherical surface or a plane.

(2) Two planes, a sphere and a plane, or two spheres intersecting at an angle ${\tfrac {\pi }{n}}$

(3) These two surfaces with a third, which may be either plane or spherical, cutting both orthogonally.

(4) These three surfaces with a fourth cutting the first two orthogonally and the third at an angle ${\tfrac {\pi }{n'}}$. Of these four surfaces one at least must be spherical.

We have already examined the first and second cases. In the first case we have a single image. In the second case we have $2n-1$ images arranged in two series in a circle which passes through the influencing point and is orthogonal to both surfaces. In the third case we have, besides these images, their images with respect to the third surface, that is, $4n-1$ images in all besides the influencing point.

In the fourth case we first draw through the influencing point a circle orthogonal to the first two surfaces, and determine on it the positions and magnitudes of the $n$ negative images and the $n-1$ positive images. Then through each of these 2$n$ points, including the influencing point, we draw a circle orthogonal to the third and fourth surfaces, and determine on it two series of images, $n'$ in each series. We shall obtain in this way, besides the influencing point, $2nn'-1$ positive and $2nn'$ negative images. These $4nn'$ points are the intersections of $n$ circles with $n'$ other circles, and these circles belong to the two systems of lines of curvature of a cyclide.

If each of these points is charged with the proper quantity of electricity, the surface whose potential is zero will consist of $n+n'$ spheres, forming two series of which the successive spheres of the first set intersect at angles ${\tfrac {\pi }{n}}$, and those of the second set at angles ${\tfrac {\pi }{n'}}$, while every sphere of the first set is orthogonal to every sphere of the second set.

Case of Two Spheres cutting Orthogonally. See Fig. IV at the end of this volume.

Fig. IV

Fig. 12

168.] Let $A$ and $B$, Fig. 12, be the centres of two spheres cutting each other orthogonally in $D$ and $D'$, and let the straight line $DD'$ cut the line of centres in $C$. Then $C$ is the image of $A$ with respect to the sphere $B$, and also the image of $B$ with respect to the sphere whose centre is $A$. If $AD=\alpha$, $BD=\beta$, then $AB={\sqrt {\alpha ^{2}+\beta ^{2}}}$, and if we place at $A,B,C$ quantities of electricity equal to $\alpha ,\beta$, and $-{\tfrac {\alpha \beta }{\sqrt {\alpha ^{2}+\beta ^{2}}}}$ respectively, then both spheres will be equipotential surfaces whose potential is unity.

We may therefore determine from this system the distribution of electricity in the following cases:

(1) On the conductor $PDQD'$ formed of the larger segments of both spheres. Its potential is 1, and its charge is

$\alpha +\beta -{\frac {\alpha \beta }{\sqrt {\alpha ^{2}+\beta ^{2}}}}=AD+BD-CD$

This quantity therefore measures the capacity of such a figure when free from the inductive action of other bodies.

The density at any point $P$ of the sphere whose centre is $A$, and the density at any point $Q$ of the sphere whose centre is $B$, are respectively

${\frac {1}{4\pi \alpha }}\left(1-\left({\frac {\beta }{BP}}\right)^{3}\right)$ and ${\frac {1}{4\pi \beta }}\left(1-\left({\frac {\alpha }{AQ}}\right)^{3}\right)$

At the points of intersection, $D,D'$, the density is zero.

If one of the spheres is very much larger than the other, the density at the vertex of the smaller sphere is ultimately three times that at the vertex of the larger sphere.

(2) The lens $P'DQ'D'$ formed by the two smaller segments of the spheres, charged with a quantity of electricity $=-{\frac {\alpha \beta }{\sqrt {\alpha ^{2}+\beta ^{2}}}}$, and acted on by points $A$ and $B$, charged with quantities $\alpha$ and $\beta$, is also at potential unity, and the density at any point is expressed by the same formulae.

(3) The meniscus $DPD'Q'$ formed by the difference of the segments charged with a quantity $\alpha$, and acted on by points $B$ and $C$, charged respectively with quantities $\beta$ and ${\frac {-\alpha \beta }{\sqrt {\alpha ^{2}+\beta ^{2}}}}$, is also in equilibrium at potential unity.

(4) The other meniscus $QDP'D'$ under the action of $A$ and $C$.

We may also deduce the distribution of electricity on the following internal surfaces.

The hollow lens $P'DQ'D$ under the influence of the internal electrified point $C$ at the centre of the circle $DD'$.

The hollow meniscus under the influence of a point at the centre of the concave surface.

The hollow formed of the two larger segments of both spheres under the influence of the three points $A,B,C$.

But, instead of working out the solutions of these cases, we shall apply the principle of electrical images to determine the density of the electricity induced at the point $P$ of the external surface of the conductor $PDQD'$ by the action of a point at $O$ charged with unit of electricity.

Let

${\begin{array}{lllllll}OA=a,&&OB=b,&&OP=r,&&BP=p,\\\\AD=\alpha ,&&BD=\beta ,&&AB={\sqrt {\alpha ^{2}+\beta ^{2}}}.\end{array}}$

Invert the system with respect to a sphere of radius unity and centre $O$.

The two spheres will remain spheres, cutting each other orthogonally, and having their centres in the same radii with $A$ and $B$. If we indicate by accented letters the quantities corresponding to the inverted system,

${\begin{array}{l}a'={\frac {a}{a^{2}-\alpha ^{2}}},\ b'={\frac {b}{b^{2}-\beta ^{2}}},\ \alpha '={\frac {\alpha }{a^{2}-\alpha ^{2}}},\ \beta '={\frac {\beta }{b^{2}-\beta ^{2}}},\\\\r'={\frac {1}{r}},\ p'^{2}={\frac {\beta ^{2}r^{2}+\left(b^{2}-\beta ^{2}\right)\left(p^{2}-\beta ^{2}\right)}{r^{2}\left(b^{2}-\beta ^{2}\right)^{2}}}.\end{array}}$

If, in the inverted system, the potential of the surface is unity, then the density at the point $P'$ is

$\sigma '={\frac {1}{4\pi \alpha '}}\left(1-\left({\frac {\beta }{p'}}\right)^{3}\right).$

If, in the original system, the density at $P$ is $\sigma$, then

${\frac {\sigma }{\sigma '}}={\frac {1}{r^{3}}},$

and the potential is ${\tfrac {1}{r}}$. By placing $O$ at a negative charge of electricity equal to unity, the potential will become zero over the surface, and the density at $P$ will be

$\sigma ={\frac {1}{4\pi }}{\frac {a^{2}-\alpha ^{2}}{\alpha r^{3}}}\left(1-{\frac {\beta ^{3}r^{3}}{\left(\beta ^{2}r^{2}+\left(b^{2}-\beta ^{2}\right)\left(p^{2}-\beta ^{2}\right)\right)^{\frac {3}{2}}}}\right)$

This gives the distribution of electricity on one of the spherical surfaces due to a charge placed at $O$. The distribution on the other spherical surface may be found by exchanging $a$ and $b$, $\alpha$ and $\beta$, and putting $q$ or $AQ$ instead of $p$.

To find the total charge induced on the conductor by the electrified point at $O$, let us examine the inverted system.

In the inverted system we have a charge $\alpha '$ at $A'$, and $\beta '$ at $B'$, and a negative charge ${\frac {\alpha '\beta '}{\sqrt {\alpha '^{2}+\beta '^{2}}}}$ at a point $C'$ in the line $A'B'$ such that

$AC:CB::\alpha '^{2}:\beta '^{2}$

If $AO'=a',\ OB'=b',\ OC'=c',$ we find

$c'^{2}={\frac {a'^{2}\beta '^{2}+b'^{2}\alpha '^{2}-\alpha '^{2}\beta '^{2}}{\alpha '^{2}+\beta '^{2}}}$

Inverting this system the charges become

${\frac {\alpha '}{a'}}={\frac {\alpha }{a}},\ {\frac {\beta '}{b'}}={\frac {\beta }{b}};$

and

$-{\frac {\alpha '\beta '}{\sqrt {\alpha {}^{2}+\beta {}^{2}}}}{\frac {1}{c'}}=-{\frac {\alpha \beta }{\sqrt {a{}^{2}\beta {}^{2}+b{}^{2}\alpha {}^{2}-\alpha {}^{2}\beta {}^{2}}}}.$

Hence the whole charge on the conductor due to a unit of negative electricity at $O$ is

${\frac {\alpha }{a}}+{\frac {\beta }{b}}-{\frac {\alpha \beta }{\sqrt {a{}^{2}\beta {}^{2}+b{}^{2}\alpha {}^{2}-\alpha {}^{2}\beta {}^{2}}}}.$

Distribution of Electricity on Three Spherical Surfaces which Intersect at Right Angles.

Fig. 13

169.] Let the radii of the spheres be $\alpha ,\beta ,\gamma$, then

$BC={\sqrt {\beta ^{2}+\gamma ^{2}}},\ CA={\sqrt {\gamma ^{2}+\alpha ^{2}}},\ AB={\sqrt {\alpha ^{2}+\beta ^{2}}}.$

Let $PQR$, Fig. 13, be the feet of the perpendiculars from $ABC$ on the opposite sides of the triangle, and let $O$ be the intersection of perpendiculars.

Then $P$ is the image of $B$ in the sphere $\gamma$, and also the image of $C$ in the sphere $\beta$. Also $O$ is the image of $P$ in the sphere $\alpha$.

Let charges $\alpha ,\beta$, and $\gamma$ be placed at $A,B,$ and $C$.

Then the charge to be placed at $P$ is

$-{\frac {\beta \gamma }{\sqrt {\beta ^{2}+\gamma ^{2}}}}=-{\frac {1}{\sqrt {{\frac {1}{\beta ^{2}}}+{\frac {1}{\gamma ^{2}}}}}}.$

Also $AP={\tfrac {\sqrt {\beta ^{2}\gamma ^{2}+\gamma ^{2}\alpha ^{2}+\alpha ^{2}\beta ^{2}}}{\sqrt {\beta ^{2}+\gamma ^{2}}}}$, so that the charge at $O$, considered as the image of $P$, is

${\frac {\alpha \beta \gamma }{\sqrt {\beta ^{2}\gamma ^{2}+\gamma ^{2}\alpha ^{2}+\alpha ^{2}\beta ^{2}}}}={\frac {1}{\sqrt {{\frac {1}{\alpha ^{2}}}+{\frac {1}{\beta ^{2}}}+{\frac {1}{\gamma ^{2}}}}}}.$

In the same way we may find the system of images which are electrically equivalent to four spherical surfaces at potential unity intersecting at right angles.

If the radius of the fourth sphere is $\delta$, and if we make the charge at the centre of this sphere =$\delta$, then the charge at the intersection of the line of centres of any two spheres, say $\alpha$ and $\beta$, with their plane of intersection, is

$-{\frac {1}{\sqrt {{\frac {1}{\alpha ^{2}}}+{\frac {1}{\beta ^{2}}}}}}$

The charge at the intersection of the plane of any three centres $ABC$ with the perpendicular from $D$ is

$+{\frac {1}{\sqrt {{\frac {1}{\alpha ^{2}}}+{\frac {1}{\beta ^{2}}}+{\frac {1}{\gamma ^{2}}}}}},$

and the charge at the intersection of the four perpendiculars is

$-{\frac {1}{\sqrt {{\frac {1}{\alpha ^{2}}}+{\frac {1}{\beta ^{2}}}+{\frac {1}{\gamma ^{2}}}+{\frac {1}{\delta ^{2}}}}}}.$

System of Four Spheres Intersecting at Right Angles under the Action of an Electrified Point.

170.] Let the four spheres be $A,B,C,D$, and let the electrified point be $O$. Draw four spheres $A_{1},B_{1},C_{1},D_{1}$, of which any one, $A_{1}$, passes through and cuts three of the spheres, in this case $B,C$, and $D$, at right angles. Draw six spheres $(ab),(ac),(ad),(be),(bd),(cd)$, of which each passes through and through the circle of intersection of two of the original spheres.

The three spheres $B_{1},C_{1},D_{1}$ will intersect in another point besides $O$. Let this point be called $A'$, and let $B',C'$, and $D'$ be the intersections of $C_{1},D_{1},A_{1}$ of $D_{1},A_{1},B_{1}$, and of $A_{1},B_{1},C_{1}$ respectively. Any two of these spheres, $A_{1},B_{1}$, will intersect one of the six ($cd$) in a point ($a'b'$). There will be six such points.

Any one of the spheres, $A_{1}$, will intersect three of the six $(ab),(ac),(ad)$ in a point $a'$. There will be four such points. Finally, the six spheres $(ab),(ac),(ad),(cd),(db),(bc)$, will intersect in one point $S$.

If we now invert the system with respect to a sphere of radius $R$ and centre $O$, the four spheres $A,B,C,D$ will be inverted into spheres, and the other ten spheres will become planes. Of the points of intersection the first four $A',B',C',D'$ will become the centres of the spheres, and the others will correspond to the other eleven points in the preceding article. These fifteen points form the image of $O$ in the system of four spheres.

At the point $A'$, which is the image of $O$ in the sphere $A$, we must place a charge equal to the image of $O$, that is, $-{\tfrac {\alpha }{a}}$, where $\alpha$ is the radius of the sphere $A$, and $a$ is the distance of its centre from $O$. In the same way we must place the proper charges at $B',C',D'$.

The charges for each of the other eleven points may be found from the expressions in the last article by substituting $\alpha ',\beta ',\gamma ',\delta '$ for $\alpha ,\beta ,\gamma ,\delta$, and multiplying the result for each point by the distance of the point from $O$, where

$\alpha '=-{\frac {\alpha }{a^{2}-\alpha ^{2}}},\ \beta '=-{\frac {\beta }{b^{2}-\beta ^{2}}},\ \gamma '=-{\frac {\gamma }{c^{2}-\gamma ^{2}}},\ \delta '=-{\frac {\delta }{d^{2}-\delta ^{2}}}.$

Two Spheres not Intersecting.

171.] When a space is bounded by two spherical surfaces which do not intersect, the successive images of an influencing point within this space form two infinite series, all of which lie beyond the spherical surfaces, and therefore fulfil the condition of the applicability of the method of electrical images.

Any two non-intersecting spheres may be inverted into two concentric spheres by assuming as the point of inversion either of the two common inverse points of the pair of spheres.

We shall begin, therefore, with the case of two uninsulated concentric spherical surfaces, subject to the induction of an electrified point placed between them.

Let the radius of the first be $b$, and that of the second be $be^{\varpi }$, and let the distance of the influencing point from the centre be $r=be^{u}$.

Then all the successive images will be on the same radius as the influencing point.

Let $Q_{0}$, Fig. 14, be the image of $P$ in the first sphere, $P_{1}$ that of $Q_{0}$ in the second sphere, $Q_{1}$ that of $P_{1}$ in the first sphere, and so on; then

Fig. 14

${\begin{array}{rll}&&OP_{s}\cdot OQ_{s}=b^{2},\\\mathrm {and} &&OP_{s}\cdot OQ_{s-1}=b^{2}e^{2\varpi },\\\mathrm {also} &&OQ_{0}=be^{-u}\\&&OP_{1}=be^{u+2\varpi },\\&&OQ_{1}=be^{-(u+2\varpi )},\ etc.\\\mathrm {Hence} &&OP_{s}=be^{(u+2s\varpi )},\\&&OQ_{s}=be^{-(u+2s\varpi )}.\end{array}}$

If the charge of $P$ is denoted by $P$, then

$P_{s}=Pe^{s\varpi },\ Q_{s}=-Pe^{-(u+s\varpi )}.$

Next, let $Q_{1}'$ be the image of $P$ in the second sphere, $P_{1}'$ that of $Q_{1}'$ in the first, &c.,

${\begin{array}{rlcrl}OQ_{1}'=&be^{2\varpi -u},&&OP_{1}'=&be^{u-2\varpi },\\OQ_{2}'=&be^{4\varpi -u},&&OP_{2}'=&be^{u-4\varpi },\\OP_{s}'=&be^{u-2s\varpi },&&OQ_{s}'=&be^{2s\varpi -u},\\P_{s}'=&Pe^{s\varpi },&&Q_{s}'=&Pe^{s\varpi -u}.\end{array}}$

Of these images all the $P's$ are positive, and all the $Q's$ negative, all the $P'$'s and $Q'$'s belong to the first sphere, and all the $P$'s and $Q$'s to the second.

The images within the first sphere form a converging series, the sum of which is

$-P{\frac {e^{\varpi -u}-1}{e^{\varpi }-1}}.$

This therefore is the quantity of electricity on the first or interior sphere. The images outside the second sphere form a diverging series, but the surface-integral of each with respect to the spherical surface is zero. The charge of electricity on the exterior spherical surface is therefore

$P\left({\frac {e^{\varpi -u}-1}{e^{\varpi }-1}}-1\right)=-P{\frac {e^{\varpi }-e^{\varpi -u}}{e^{\varpi }-1}}$

If we substitute for these expressions their values in terms of $OA,OB$, and $OP$, we find

charge on $A=-P{\frac {OA}{OP}}{\frac {PB}{AB}},$

charge on $B=-P{\frac {OB}{OP}}{\frac {AP}{AB}}.$

If we suppose the radii of the spheres to become infinite, the case becomes that of a point placed between two parallel planes $A$ and $B$. In this case these expressions become

charge on $A=-P{\frac {PB}{AB}},$,

charge on $B=-P{\frac {AP}{AB}}.$.

Fig. 15

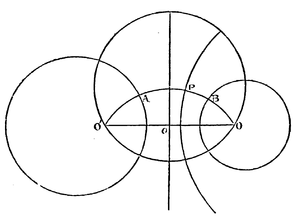
172.] In order to pass from this case to that of any two spheres not intersecting each other, we begin by finding the two common inverse points $O,O'$ through which all circles pass that are orthogonal to both spheres. Then, inverting the system with respect to either of these points, the spheres become concentric, as in the first case.

The radius $OAPB$ on which the successive images lie becomes an arc of a circle through $O$ and $O'$, and the ratio of $O'P$ to $OP$ is equal to $Ce^{u}$ where $C$ is a numerical quantity which for simplicity we may make equal to unity.

We therefore put

$u=\log {\frac {O'P}{OP}},\ \alpha =\log {\frac {O'A}{OA}},\ \beta =\log {\frac {O'B}{OB}}.$

Let

$\beta -\alpha =\varpi ,\ u-a=\theta .$

Then all the successive images of $P$ will lie on the arc $OAPBO'$.

The position of the image of $P$ in $A$ is $Q_{0}$ where

$u\left(Q_{0}\right)=\log {\frac {O'Q}{OQ}}=2a-u.$

That of $Q_{0}$ in $B$ is $P_{1}$ where

$u\left(P_{1}\right)=\log {\frac {O'P_{1}}{OP_{1}}}=u+2\varpi .$

Similarly

$u\left(P_{s}\right)=u+2s\varpi ,\ u\left(Q_{s}\right)=2a-u-2s\varpi .$

In the same way if the successive images of $P$ in $B,A,B$, &c. are $Q_{0}',P_{1}',Q_{1}',$, &c.,

${\begin{array}{lll}u\left(Q_{0}'\right)=2\beta -u,&&u\left(P_{0}'\right)=u-2\varpi ;\\u\left(P_{s}'\right)=u-2s\varpi ,&&u\left(Q_{s}'\right)=2\beta -u+2s\varpi .\end{array}}$

To find the charge of any image $P_{s}$ we observe that in the inverted figure its charge is

$P{\sqrt {\frac {OP_{s}}{OP}}}$

In the original figure we must multiply this by $O'P_{s}$. Hence the charge of $P_{s}$ in the dipolar figure is

$P{\sqrt {\frac {OP_{s}\cdot O'P_{s}}{OP\cdot O'P}}}.$

If we make $\xi ={\sqrt {OP\cdot O'P}}$, and call $\xi$ the parameter of the point $P$, then we may write

$P_{s}={\frac {\xi _{s}}{\xi }}P,$

or the charge of any image is proportional to its parameter.

If we make use of the curvilinear coordinates $u$ and $v$, such that

$e^{u+{\sqrt {-1}}v}={\frac {x+{\sqrt {-1}}y-k}{x+{\sqrt {-1}}y+k}},$

then

$x=-{\frac {k\sin hu}{\cos hu-\cos v}},\ y={\frac {k\sin v}{\cos hu-\cos v}};$

$x^{2}+(y-k\cot v)^{2}=k^{2}\operatorname {\{} cosec\}^{2}v,$

$(x+k\cot hu)^{2}+y^{2}=k^{2}\operatorname {\{} cosec\}h^{2}u,$

$\cot v={\frac {x^{2}+y^{2}-k^{2}}{2ky}},\ \cot hu=-{\frac {x^{2}+y^{2}+k^{2}}{2kx}};$

$\xi ={\frac {\sqrt {2k}}{\sqrt {\cos hu-\cos v}}}.$^[2]

Since the charge of each image is proportional to its parameter, $\xi$, and is to be taken positively or negatively according as it is of the form $P$ or $Q$, we find

${\begin{array}{ll}P_{s}=&{\frac {P{\sqrt {\cos hu-\cos v}}}{\sqrt {\cos h(u+2s\varpi )-\cos v}}},\\\\Q_{s}=-&{\frac {P{\sqrt {\cos hu-\cos v}}}{\sqrt {\cos h(2\alpha -u-2s\varpi )-\cos v}}},\\\\P'_{s}=&{\frac {P{\sqrt {\cos hu-\cos v}}}{\sqrt {\cos h(u-2s\varpi )-\cos v}}},\\\\Q_{s}=-&{\frac {P{\sqrt {\cos hu-\cos v}}}{\sqrt {\cos h(2\beta -u+2s\varpi )-\cos v}}}.\end{array}}$

We have now obtained the positions and charges of the two infinite series of images. We have next to determine the total charge on the sphere $A$ by finding the sum of all the images within it which are of the form $Q$ or $P'$. We may write this

$P{\sqrt {\cos hu-\cos v}}\sum _{s=1}^{s=\infty }{\frac {1}{\sqrt {\cos h(u-2s\varpi )-\cos v}}},$ $-P{\sqrt {\cos hu-\cos v}}\sum _{s=0}^{s=\infty }{\frac {1}{\sqrt {\cos h(2\alpha -u-2s\varpi )-\cos v}}},$

In the same way the total induced charge on $B$ is

$P{\sqrt {\cos hu-\cos v}}\sum _{s=1}^{s=\infty }{\frac {1}{\sqrt {\cos h(u+2s\varpi )-\cos v}}},$ $-P{\sqrt {\cos hu-\cos v}}\sum _{s=1}^{s=\infty }{\frac {1}{\sqrt {\cos h(2\beta -u+2s\varpi )-\cos v}}},$

173.] We shall apply these results to the determination of the coefficients of capacity and induction of two spheres whose radii are $a$ and $b$, and the distance of whose centres is $c$.

In this case

$k={\frac {\sqrt {a^{4}+b^{4}+c^{4}-2b^{2}c^{2}-2c^{2}a^{2}-2a^{2}b^{2}}}{2c}};$ $\sin h\cdot \alpha ={\frac {k}{a}},\ \sin h\cdot \beta ={\frac {k}{b}}.$

Let the sphere $A$ be at potential unity, and the sphere $B$ at potential zero.

Then the successive images of a charge $a$ placed at the centre of the sphere $A$ will be those of the actual distribution of electricity. All the images will lie on the axis between the poles and the centres of the spheres.

The values of $u$ and $v$ for the centre of the sphere $A$ are

$u=2\alpha ,\ v=0.$

Hence we must substitute $a$ or $k{\tfrac {1}{\sin h\alpha }}$ for $P$, and 2$a$ for $u$, and $v=0$ in the equations, remembering that $P$ itself forms part of the charge of $A$. We thus find for the coefficient of capacity of $A$

$q_{aa}=k\sum _{s=0}^{s=\infty }{\frac {1}{\sin h(s\varpi -\alpha )}},$

for the coefficient of induction of $A$ on $B$ or of $B$ on $A$

$q_{ab}=-k\sum _{s=1}^{s=\infty }{\frac {1}{\sin hs\varpi }},$

and for the coefficient of capacity of $B$

$q_{bb}=k\sum _{s=1}^{s=\infty }{\frac {1}{\sin h(\beta +s\varpi )}}.$

To calculate these quantities in terms of $a$ and $b$, the radii of the spheres, and of $c$ the distance between their centres, we make use of the following quantities

$p=e^{\alpha }={\sqrt {{\frac {k^{2}}{a^{2}}}+1}}-{\frac {k}{a}},$ $q=e^{\beta }={\sqrt {{\frac {k^{2}}{b^{2}}}+1}}+{\frac {k}{b}},$ ${\frac {q}{p}}=r=e^{\varpi }=\left({\sqrt {{\frac {k^{2}}{a^{2}}}+1}}+{\frac {k}{a}}\right)\left({\sqrt {{\frac {k^{2}}{b^{2}}}+1}}+{\frac {k}{b}}\right).$

We may now write the hyperbolic sines in terms of $p,q,r$; thus

${\begin{array}{ll}q_{aa}=&\sum _{s=0}^{s=\infty }{\frac {2k}{{\frac {r^{s}}{p}}-{\frac {p}{r^{s}}}}},\\q_{ab}=-&\sum _{s=1}^{s=\infty }{\frac {2k}{r^{s}-{\frac {1}{r^{s}}}}},\\\\q_{bb}=&\sum _{s=0}^{s=\infty }{\frac {2k}{qr^{s}-{\frac {1}{qr^{s}}}}}.\end{array}}$

Proceeding to the actual calculation we find, either by this process or by the direct calculation of the successive images as shewn in Sir W. Thomson's paper, which is more convenient for the earlier part of the series,

${\begin{array}{l}q_{aa}=a+{\frac {a^{2}b}{c^{2}-b^{2}}}+{\frac {a^{3}b^{2}}{\left(c^{2}-b^{2}+ac\right)\left(c^{2}-b^{2}-ac\right)}}+etc.,\\\\q_{ab}=-{\frac {ab}{c}}-{\frac {a^{2}b^{2}}{c\left(c^{2}-a^{2}-b^{2}\right)}}-{\frac {a^{3}b^{3}}{c\left(c^{2}-a^{2}-b^{2}+ab\right)\left(c^{2}-a^{2}-b^{2}-ab\right)}}-etc.\\\\q_{bb}=b+{\frac {ab^{2}}{c^{2}-a^{2}}}+{\frac {a^{2}b^{3}}{\left(c^{2}-a^{2}+bc\right)\left(c^{2}-a^{2}-bc\right)}}+etc.\end{array}}$

174.] We have then the following equations to determine the charges $E_{a}$ and $E_{b}$ of the two spheres when electrified to potentials $V_{a}$ and $V_{b}$ respectively,

$E_{a}=V_{a}q_{aa}+V_{b}q_{ab},$ $E_{b}=V_{a}q_{ab}+V_{b}q_{bb}.$

If we put

$q_{aa}q_{bb}-q_{ab}^{2}=D={\frac {1}{D'}},$

and

$p_{aa}=q_{bb}D',\ p_{ab}=-q_{ab}D',\ p_{bb}=q_{aa}D',$

whence

$p_{aa}p_{bb}-p_{ab}^{2}=D';$

then the equations to determine the potentials in terms of the charges are

$V_{a}=p_{aa}E_{a}+p_{ab}E_{b},$ $V_{b}=p_{ab}E_{a}+p_{bb}E_{b},$

and $p_{aa},p_{ab}$, and $p_{bb}$ are the coefficients of potential.

The total energy of the system is, by Art. 85,

${\begin{array}{ll}Q&={\frac {1}{2}}\left(E_{a}V_{a}+E_{b}V_{b}\right),\\\\&={\frac {1}{2}}\left(V_{a}^{2}q_{aa}+2V_{a}V_{b}q_{ab}+V_{b}^{2}q_{bb}\right),\\\\&={\frac {1}{2}}\left(E_{a}^{2}p_{aa}+2E_{a}E_{b}p_{ab}+E_{b}^{2}p_{bb}\right).\end{array}}$

The repulsion between the spheres is therefore, by Arts. 92, 93,

${\begin{array}{ll}F=&{\frac {1}{2}}\left\{V_{a}^{2}{\frac {dq_{aa}}{dc}}+2V_{a}V_{b}{\frac {dq_{ab}}{dc}}+V_{b}^{2}{\frac {dq_{bb}}{dc}}\right\},\\\\=-&{\frac {1}{2}}\left\{E_{a}^{2}{\frac {dp_{aa}}{dc}}+2E_{a}E_{b}{\frac {dp_{ab}}{dc}}+E_{b}^{2}{\frac {dp_{bb}}{dc}}\right\},\end{array}}$

where $c$ is the distance between the centres of the spheres.

Of these two expressions for the repulsion, the first, which expresses it in terms of the potentials of the spheres and the variations of the coefficients of capacity and induction, is the most convenient for calculation.

We have therefore to differentiate the $q$'s with respect to $c$. These quantities are expressed as functions of $k,\alpha ,\beta$, and $\varpi$, and must be differentiated on the supposition that $a$ and $b$ are constant. From the equations

$k=a\sin ha=b\sin h\beta =c{\frac {\sin ha\sin h\beta }{\sin h\varpi }},$

we find

${\begin{array}{l}{\frac {d\alpha }{dc}}={\frac {\sin ha\cos h\beta }{k\sin h\varpi }},\\\\{\frac {d\beta }{dc}}={\frac {\sin ha\sin h\beta }{k\sin h\varpi }},\\\\{\frac {d\varpi }{dc}}={\frac {1}{k}},\\\\{\frac {dk}{dc}}={\frac {\cos ha\cos h\beta }{\sin h\varpi }};\end{array}}$

whence we find

${\begin{array}{l}{\frac {dq_{aa}}{dc}}={\frac {\cos ha\cos h\beta }{\sin h\varpi }}{\frac {q_{aa}}{k}}-\sum _{s=0}^{s=\infty }{\frac {(sc-a\cos h\beta )\cos h(s\varpi -\alpha )}{c(\sin h(s\varpi -\alpha ))^{2}}},\\\\{\frac {dq_{ab}}{dc}}={\frac {\cos ha\cos h\beta }{\sin h\varpi }}{\frac {q_{ab}}{k}}+\sum _{s=1}^{s=\infty }{\frac {s\cos hs\varpi }{(\sin hs\varpi )^{2}}},\\\\{\frac {dq_{bb}}{dc}}={\frac {\cos ha\cos h\beta }{\sin h\varpi }}{\frac {q_{bb}}{k}}-\sum _{s=0}^{s=\infty }{\frac {(sc+b\cos h\alpha )\cos h(\beta +s\varpi )}{c(\sin h(\beta +s\varpi ))^{2}}}.\end{array}}$

Sir William Thomson has calculated the force between two spheres of equal radius separated by any distance less than the diameter of one of them. For greater distances it is not necessary to use more than two or three of the successive images.

The series for the differential coefficients of the $q$'s with respect to $c$ are easily obtained by direct differentiation

${\begin{array}{l}{\frac {dq_{aa}}{dc}}=-{\frac {2a^{2}bc}{\left(c^{2}-b^{2}\right)^{2}}}-{\frac {2a^{3}b^{2}c\left(2c^{2}-2b^{2}-a^{2}\right)}{\left(c^{2}-b^{2}+ac\right)^{2}\left(c^{2}-b^{2}-ac\right)^{2}}}-etc.,\\\\{\frac {dq_{ab}}{dc}}={\frac {ab}{c^{2}}}+{\frac {a^{2}b^{2}\left(3c^{2}-a^{2}-b^{2}\right)}{c^{2}\left(c^{2}-a^{2}-b^{2}\right)}}+{\frac {a^{3}b^{3}\left\{\left(5c^{2}-a^{2}-b^{2}\right)\left(c^{2}-a^{2}-b^{2}\right)-a^{2}b^{2}\right\}}{c^{2}\left(c^{2}-a^{2}-b^{2}+ab\right)^{2}\left(c^{2}-a^{2}-b^{2}-ab\right)^{2}}}-etc.,\\\\{\frac {dq_{bb}}{dc}}=-{\frac {2ab^{2}c}{\left(c^{2}-b^{2}\right)^{2}}}-{\frac {2a^{2}b^{3}c\left(2c^{2}-2a^{2}-b^{2}\right)}{\left(c^{2}-a^{2}+bc\right)^{2}\left(c^{2}-a^{2}-bc\right)^{2}}}-etc.\end{array}}$

Distribution of Electricity on Two Spheres in Contact.

175.] If we suppose the two spheres at potential unity and not influenced by any other point, then, if we invert the system with respect to the point of contact, we shall have two parallel planes, distant ${\tfrac {1}{2a}}$ and ${\tfrac {1}{2b}}$ from the point of inversion, and electrified by the action of a unit of electricity at that point.

There will be a series of positive images, each equal to unity, at distances $s\left({\tfrac {1}{a}}+{\tfrac {1}{b}}\right)$ from the origin, where $s$ may have any integer value from $-\infty$ to $+\infty$.

There will also be a series of negative images each equal to -1, the distances of which from the origin, reckoned in the direction of $a$, are ${\tfrac {1}{a}}+s\left({\tfrac {1}{a}}+{\tfrac {1}{b}}\right)$.

When this system is inverted back again into the form of the two spheres in contact, we have a corresponding series of negative images, the distances of which from the point of contact are of the form ${\tfrac {1}{s\left({\frac {1}{a}}+{\frac {1}{b}}\right)}}$, where $s$ is positive for the sphere $A$ and negative for the sphere $B$. The charge of each image, when the potential of the spheres is unity, is numerically equal to its distance from the point of contact, and is always negative.

There will also be a series of positive images whose distances from the point of contact measured in the direction of the centre of $a$, are of the form ${\tfrac {1}{{\frac {1}{a}}+s\left({\frac {1}{a}}+{\frac {1}{b}}\right)}}$.

When $s$ is zero, or a positive integer, the image is in the sphere $A$.

When $s$ is a negative integer the image is in the sphere $B$.

The charge of each image is measured by its distance from the origin and is always positive.

The total charge of the sphere $A$ is therefore

$E_{a}=\sum _{s=0}^{s=\infty }{\frac {1}{{\frac {1}{a}}+s\left({\frac {1}{a}}+{\frac {1}{b}}\right)}}-{\frac {ab}{a+b}}\sum _{s=1}^{s=\infty }{\frac {1}{s}}.$

Each of these series is infinite, but if we combine them in the form

$E_{a}=\sum _{s=1}^{s=\infty }{\frac {a^{2}b}{s(a+b)(s(a+b)-a)}}$

the series becomes converging.

In the same way we find for the charge of the sphere $B$,

${\begin{array}{ll}E_{b}&=\sum _{s=1}^{s=\infty }{\frac {ab}{s(a+b)-b}}-{\frac {ab}{a+b}}\sum _{s=-1}^{s=-\infty }{\frac {1}{s}},\\\\&=\sum _{s=1}^{s=\infty }{\frac {ab^{2}}{s(a+b)\{s(a+b)-b\}}}.\end{array}}$

The values of $E_{a}$ and $E_{b}$ are not, so far as I know, expressible in terms of known functions. Their difference, however, is easily expressed, for

${\begin{array}{ll}E_{a}-E_{b}&=\sum _{s=-\infty }^{s=\infty }{\frac {ab}{b+s(a+b)}},\\\\&={\frac {\pi ab}{a+b}}\cot {\frac {\pi b}{a+b}}.\end{array}}$

When the spheres are equal the charge of each for potential unity is

${\begin{array}{ll}E_{a}&=a\sum _{s=1}^{s=\infty }{\frac {1}{2s(2s-1)}}\\\\&=a\left(1-{\frac {1}{2}}+{\frac {1}{3}}-{\frac {1}{4}}+etc.\right),\\\\&=a\log _{e}2=1.0986a.\end{array}}$

When the sphere $A$ is very small compared with the sphere $B$ the charge on $A$ is

$E_{a}={\frac {a^{2}}{b}}\sum _{s=1}^{s=\infty }{\frac {1}{s^{2}}}$ approximately;

or $E_{a}={\frac {\pi ^{2}}{6}}{\frac {a^{2}}{b}}.$

The charge on $B$ is nearly the same as if $A$ were removed, or

$E_{b}=b.$

The mean density on each sphere is found by dividing the charge by the surface. In this way we get

${\begin{array}{l}\sigma _{a}={\frac {E_{a}}{4\pi a^{2}}}={\frac {\pi }{24b}}\\\\\sigma _{b}={\frac {E_{b}}{4\pi b^{2}}}={\frac {1}{4\pi b}},\\\\\sigma _{a}={\frac {\pi ^{2}}{6}}\sigma _{b}.\end{array}}$

Hence, if a very small sphere is made to touch a very large one, the mean density on the small sphere is equal to that on the large sphere multiplied by ${\tfrac {\pi ^{2}}{6}}$, or 1.644936.

Application of Electrical Inversion to the case of a Spherical Bowl.

176.] One of the most remarkable illustrations of the power of Sir W. Thomson's method of Electrical Images is furnished by his investigation of the distribution of electricity on a portion of a spherical surface bounded by a small circle. The results of this investigation, without proof, were communicated to M. Liouville and published in his Journal in 1847. The complete investigation is given in the reprint of Thomson's Electrical Papers, Article XV. I am not aware that a solution of the problem of the distribution of electricity on a finite portion of any curved surface has been given by any other mathematician.

As I wish to explain the method rather than to verify the calculation, I shall not enter at length into either the geometry or the integration, but refer my readers to Thomson's work.

Distribution of Electricity on an Ellipsoid.

177.] It is shewn by a well-known method^[3] that the attraction of a shell bounded by two similar and similarly situated and concentric ellipsoids is such that there is no resultant attraction on any point within the shell. If we suppose the thickness of the shell to diminish indefinitely while its density increases, we ultimately arrive at the conception of a surface-density varying as the perpendicular from the centre on the tangent plane, and since the resultant attraction of this superficial distribution on any point within the ellipsoid is zero, electricity, if so distributed on the surface, will be in equilibrium.

Hence, the surface-density at any point of an ellipsoid undisturbed by external influence varies as the distance of the tangent plane from the centre.

Distribution of Electricity on a Disk.

By making two of the axes of the ellipsoid equal, and making the third vanish, we arrive at the case of a circular disk, and at an expression for the surface-density at any point $P$ of such a disk when electrified to the potential $V$ and left undisturbed by external influence. If $\sigma$ be the surface-density on one side of the disk, and if $KPL$ be a chord drawn through the point $P$, then

$\sigma ={\frac {V}{2\pi ^{2}{\sqrt {KP\cdot PL}}}}.$

Application of the Principle of Electric Inversion.

178.] Take any point $Q$ as the centre of inversion, and let $R$ be the radius of the sphere of inversion. Then the plane of the disk becomes a spherical surface passing through $Q$${\displaystyle }$, and the disk itself becomes a portion of the spherical surface bounded by a circle. We shall call this portion of the surface the bowl.

If $S'$ is the disk electrified to potential $V'$ and free from external influence, then its electrical image $S$ will be a spherical segment at potential zero, and electrified by the influence of a quantity $V'R$ of electricity placed at $Q$.

We have therefore by the process of inversion obtained the solution of the problem of the distribution of electricity on a bowl or a plane disk when under the influence of an electrified point in the surface of the sphere or plane produced.

Influence of an Electrified Point placed on the unoccupied part of the Spherical Surface.

The form of the solution, as deduced by the principles already given and by the geometry of inversion, is as follows:

If $C$ is the central point or pole of the spherical bowl $S$, and if $a$ is the distance from $C$ to any point in the edge of the segment, then, if a quantity $q$ of electricity is placed at a point $Q$ in the surface of the sphere produced, and if the bowl $S$ is maintained at potential zero, the density $\sigma$ at any point $P$ of the bowl will be

$\sigma ={\frac {1}{2\pi ^{2}}}{\frac {q}{QP^{2}}}{\sqrt {\frac {CQ^{2}-a^{2}}{a^{2}-CP^{2}}}},$

$CQ,CP$, and $QP$ being the straight lines joining the points, $C,Q$, and $P$.

It is remarkable that this expression is independent of the radius of the spherical surface of which the bowl is a part. It is therefore applicable without alteration to the case of a plane disk.

Influence of any Number of Electrified Points.

Now let us consider the sphere as divided into two parts, one of which, the spherical segment on which we have determined the electric distribution, we shall call the bowl, and the other the remainder, or unoccupied part of the sphere on which the influencing point $Q$ is placed.

If any number of influencing points are placed on the remainder of the sphere, the electricity induced by these on any point of the bowl may be obtained by the summation of the densities induced by each separately.

179.] Let the whole of the remaining surface of the sphere be uniformly electrified, the surface-density being $\rho$, then the density at any point of the bowl may be obtained by ordinary integration over the surface thus electrified.

We shall thus obtain the solution of the case in which the bowl is at potential zero, and electrified by the influence of the remaining portion of the spherical surface rigidly electrified with density $\rho$.

Now let the whole system be insulated and placed within a sphere of diameter $f$, and let this sphere be uniformly and rigidly electrified so that its surface-density is $\rho '$.

There will be no resultant force within this sphere, and therefore the distribution of electricity on the bowl will be unaltered, but the potential of all points within the sphere will be increased by a quantity $V$ where

$V={\frac {2\pi \rho '}{f}}.$

Hence the potential at every point of the bowl will now be $V$.

Now let us suppose that this sphere is concentric with the sphere of which the bowl forms a part, and that its radius exceeds that of the latter sphere by an infinitely small quantity.

We have now the case of the bowl maintained at potential $V$ and influenced by the remainder of the sphere rigidly electrified with superficial density $\rho +\rho '$.

180.] We have now only to suppose $\rho +\rho '=0$, and we get the case of the bowl maintained at potential $V$ and free from external influence.

If $\sigma$ is the density on either surface of the bowl at a given point when the bowl is at potential zero, and is influenced by the rest of the sphere electrified to density $\rho$, then, when the bowl is maintained at potential $V$, we must increase the density on the outside of the bowl by $\rho '$, the density on the supposed enveloping sphere.

The result of this investigation is that if $f$ is the diameter of the sphere, $a$ the chord of the radius of the bowl, and $r$ the chord of the distance of $P$ from the pole of the bowl, then the surface-density $\sigma$ on the inside of the bowl is

$\sigma ={\frac {V}{2\pi ^{2}f}}\left\{{\sqrt {\frac {f^{2}-a^{2}}{a^{2}-r^{2}}}}-\tan ^{-1}{\sqrt {\frac {f^{2}-a^{2}}{a^{2}-r^{2}}}}\right\},$

and the surface-density on the outside of the bowl at the same point is

$\sigma +{\frac {V}{2\pi f}}.$

In the calculation of this result no operation is employed more abstruse than ordinary integration over part of a spherical surface. To complete the theory of the electrification of a spherical bowl we only require the geometry of the inversion of spherical surfaces.

181.] Let it be required to find the surface-density induced at any point of the bowl by a quantity $q$ of electricity placed at a point $Q$, not now in the spherical surface produced.

Invert the bowl with respect to $Q$, the radius of the sphere of inversion being $R$. The bowl $S$ will be inverted into its image $S'$, and the point $P$ will have $P'$ for its image. We have now to determine the density $\sigma '$ at $P'$ when the bowl $S'$ is maintained at potential $V'$, such that $q=V'R$, and is not influenced by any external force.

The density $\sigma$ at the point $P$ of the original bowl is then

$\sigma =-{\frac {\sigma 'R^{3}}{QP^{3}}},$

this bowl being at potential zero, and influenced by a quantity $q$ of electricity placed at $Q$.

The result of this process is as follows:

Fig. 16.

Let the figure represent a section through the centre, $O$, of the sphere, the pole, $C$, of the bowl, and the influencing point $Q$. $D$ is a point which corresponds in the inverted figure to the unoccupied pole of the rim of the bowl, and may be found by the following construction.

Draw through $Q$ the chords $EQE'$ and $FQF'$, then if we suppose the radius of the sphere of inversion to be a mean proportional between the segments into which a chord is divided at $Q$, $E'F'$ will be the image of $EF$. Bisect the arc $F'CE'$ in $D'$, so that $F'D'=D'E'$, and draw $D'QD$ to meet the sphere in $D$. $D$ is the point required. Also through $O$, the centre of the sphere, and $Q$ draw $HOQH'$ meeting the sphere in $H$ and $H'$. Then if $P$ be any point in the bowl, the surface-density at $P$ on the side which is separated from $Q$ by the completed spherical surface, induced by a quantity $q$ of electricity at $Q$, will be

$\sigma ={\frac {q}{2\pi ^{2}}}{\frac {QH\cdot QH'}{HH'\cdot PQ^{3}}}\left\{{\frac {PQ}{DQ}}\left({\frac {CD^{2}-a^{2}}{a^{2}-CP^{2}}}\right)^{\frac {1}{2}}-\tan ^{-1}\left[{\frac {PQ}{DQ}}\left({\frac {CD^{2}-a^{2}}{a^{2}-CP^{2}}}\right)^{\frac {1}{2}}\right]\right\},$

where $a$ denotes the chord drawn from $C$, the pole of the bowl, to the rim of the bowl.

On the side next to $Q$ the surface-density is

$\sigma +{\frac {q}{2\pi ^{2}}}{\frac {QH\cdot QH'}{HH'\cdot PQ^{3}}}.$

---

1. See Thomson and Tait's Natural Philosophy, § 515.
2. In these expressions we must remember that

   $2\cos hu=e^{u}+e^{-u},\ 2\sin hu=e^{u}-e^{-u},$

   and the other functions of $u$ are derived from these by the same definitions as the corresponding trigonometrical functions.
   The method of applying dipolar coordinates to this case was given by Thomson in Liouville's Journal for 1847. See Thomson's reprint of Electrical Papers, 211, 212. In the text I have made use of the investigation of Prof. Betti, Nuovo Cimento, vol. xx, for the analytical method, but I have retained the idea of electrical images as used by Thomson in his original investigation, Phil. Mag., 1853.

3. Thomson and Tait's Natural Philosophy, § 520, or Art. 150 of this book.