A Dictionary of Music and Musicians/Helmholtz, Hermann

by Edward John Payne

HELMHOLTZ, Hermann Ludwig Ferdinand, was born Aug. 31, 1821, at Potsdam. His father was Professor at the Gymnasium there, and his mother, Caroline Penn, belonged to an emigrated English family. He studied medicine in Berlin in 1839, and rose to be Teacher of Anatomy at the Berlin Academy in 1848. In the following year he became Professor of Physiology at Königsberg; in 1858, Professor of the same at Heidelberg, and Geheimrath. In 1871 he returned to the Berlin University as Professor of Natural Philosophy, and at Christmas, 1877, was elected Eector. His essay on the Conservation of Force ('Erhaltung der Kraft") appeared in 1847; his Physiological Optics ('Physiologische Optik') in 1856–66; and his Popular Scientific Lectures ('Pop. wissensch. Vorträge') at Brunswick, 1865–76. It is, however, with his 'Treatise on the Sensations of Tone as a physiological basis for the theory of Music,'^[1] and with his valuable inventions and discoveries in relation to the art, that we are here concerned.

Professor Helmholtz has invented a double harmonium with 24 vibrators to the octave, by means of which the musician can modulate into all keys quite as easily as on a single manual tuned by equal temperament, and without the dissonant thirds and sixths which that mode of tuning introduces. The system may be easily applied to the organ and piano. It is extremely simple, as it does not add to the number of notes in the scale, and requires no new system of fingering to be learnt by the performer. This invention, originally suggested by the extremely unpleasant effect of the equally tempered harmonium, may not impossibly revolutionise modern musical practice, extending as it does to manual instruments that perfect intonation which has hitherto been attainable only by stringed instruments and the human voice. The following may be selected, amongst many others, to illustrate the nature of the discoveries of Helmholtz:—

1. Quality of Musical sounds determined by Harmonics. By means of a series of resonators, each of which on being applied to the ear reinforces any harmonic of equal pitch which may be present in a given note, Helmholtz has effected the most complete analysis of musical tone hitherto attained. The resonator is a hollow sphere of glass or metal, with two openings opposite to each other, one of which is funnel-shaped, for insertion into the ear. Let the note of the resonator be upper C, the air contained in it will vibrate very powerfully when that note is given by the voice or any musical instrument; and less powerfully when the note given is one of those lower notes which are harmonic sub-tones of C, or is, in other words, a note among the harmonics of which the upper C occurs.

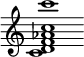	Resonator
	Harmonic sub-tones.

The chief results of Helmholtz's experiments with resonators have been given under the head Harmonics.

More curious is his determination of the nature of the vowel sounds of the human voice, in which Helmholtz has developed the discoveries of Wheatstone. The shape of the mouth-cavity is altered for the production of each particular vowel; and in each of the shapes which it assumes it may be considered as a musical instrument yielding a different note, and in the case of the compound vowels, yielding simultaneously two separate notes of different pitch, just as the neck and body of a glass bottle do. The natural resonance of the mouth-cavity, independently of the tension of the vocal chords, for different vowels, is as follows (the pronunciation of the vowels being not English but German):—

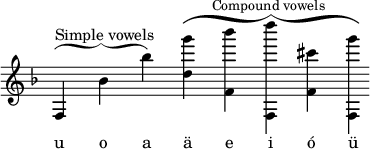
Thus, when the mouth-cavity is found to utter the sound u (oo), it is in effect a musical instrument, the natural pitch of which is lower f, and so on.

For the highly interesting experiments on vowel-pitch by means of the resonators, and the importance to singers and composers of the results deducible from them, the reader must be referred to Helmholtz's work (Ellis's translation, pp. 153–172).

2. Summational Tones. The fact that when two notes are sounded together they generate a third and deeper tone, whose vibrational number equals the difference of their several vibrational numbers, has been known to violinists ever since the time of Tartini. [See Tartini's Tones.] These tones Helmholtz calls differential tones, to distinguish them from another set of generated tones discovered by himself, the vibrational numbers of which equal the sum of the vibrational numbers of the generating tones, and which he hence calls summational tones. These tones are of course higher than the generating tones. Thus, if the chords in minims in the following figure be played forte on the violin, the double series of combinational tones above and below will be produced:—

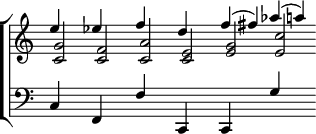	Summational tones.
	Generating tones
	Differential or Tartini's tones.

The summational tones are too weak to be distinguished by the unaided ear: while the differential tones are on some instruments intrusively audible. (In fact the violin player obtains perfect fifths on his strings by tuning until he hears the octave below the lower string.) The summational tones of the two last chords lie between F and F♯ and A♭ and A respectively.

3. Physiology of the Minor Chord. Among the most interesting of these discoveries is the reason of the heavy and quasi-dissonant effect produced by minor triads. Just intonation deepens the well-known grave, obscure, and mysterious character which belongs to minor chords; and the observations of Helmholtz on accurately tuned instruments have enabled him to trace this grave and obscure character to the presence of certain deep combinational tones, foreign to the chord, which are absent from major chords, and which without being near enough to beat, and thus actually to disturb the harmony, make themselves sufficiently audible, at least to a practised ear, as not belonging to the harmony. No minor chord can be obtained perfectly free from such false combinational tones. For the ordinary hearer the presence of these tones gives to the chord its well-known, obscure, and mysterious character, for which he is unable to account, because the weak combinational tones on which it depends are concealed by other louder tones. The fact that this unsatisfactory though not dissonant effect of the minor chord is deepened when the chord is played perfectly in tune, led musicians who wrote before the era of equal temperament to avoid the minor chord as a close, and to reserve the effect produced by minor chords for distinct passages or episodes in the composition, instead of using them in indiscriminate combination with major chords, as is the practice of ordinary modern composers. The 'Ave verum' of Mozart, and the choral hymn of Mendelssohn,^[2] 'Vaterland in deinen Gauen,' are good examples of this separation of major and minor effects as instinctively practised by the best writers.

4. Perception of musical tones by the human ear. Starting from the anatomical discoveries of the Marchese Corti, Helmholtz has shown how different parts of the ear are set in vibration by tones of different pitch. The human cochlea contains about 3000 of the rods or fibres known as 'Corti's arches.' The human ear, in fact, is a highly sensitive musical instrument, furnished with 3000 strings, which are set in motion by the concurrent vibration of external sonorous bodies, exactly in the same way in which the 'resonator' responds to a musical sound, or in which the strings of a silent violoncello or pianoforte are set in vibration by the production, in a sufficient degree of strength, of notes of equivalent pitch on any other instrument placed near it. On the perfect or imperfect anatomical constitution of these 3000 musical strings, and on their connection with the brain, depends the capacity in the human subject for the sensation of tune: probably in persons who have 'no ear' they are imperfectly developed. Deducting 200 for tones which lie beyond musical limits, there remain 2,800 for the seven octaves of musical pitch, that is, 400 for every octave. If the experiments of E. H. Weber are correct, sensitive and practised musicians can perceive a difference of pitch for which the vibrational numbers are as 1000 to 1001. Intervals so fine, falling between the pitch of two of Corti's arches, would probably set both arches unequally in vibration, that one vibrating most strongly which is nearest to the pitch of the tone.

5. Distribution of harmonic intervals. The common rule of avoiding close intervals in the bass, and of distributing intervals with tolerable evenness between the extreme tones, has long been arrived at by experience. Helmholtz has demonstrated its physiological basis to consist in the dissonant combinational tones which result from intervals otherwise distributed.

For Professor Helmholtz's deduction of other rules of musical science from the physical nature of musical sounds, together with his historical exposition of the growth of melodic scales and of modern harmony, the reader is referred to his work, as already cited.

[ E. J. P.] ]

1. 'Die Lehre von den Tonempfindungen als physiologische Grundlage für die Theorie der Musik.' Brunswick, 1863.
2. From the Festgesang for the Printing Festival.