A Dictionary of Music and Musicians/Tetrachord
TETRACHORD (Gr. τετράχορδoν). A system of four sounds, comprised within the limits of a Perfect Fourth.
It was for the purpose of superseding the cumbrous machinery of the Tetrachords upon which the old Greek Scale depended for its existence,[1]that Guido d'Arezzo invented the series of Hexachords, which, universally accepted by the Polyphonic Composers of the Middle Ages, remained in constant use until the Ecclesiastical Modes were finally abandoned in favour of our present Scale;[2] and it is only by comparing these Hexachords with the divisions of the older system that their value can be truly appreciated. It is not pretended that they were perfect; but modern mathematical science has proved that the step taken by Guido was wholly in the right direction. The improvement which led to its abandonment was, in the first instance, a purely empirical one; though we now know that it rests upon a firm mathematical basis. The natural craving of the refined musical ear for a Leading Note led, first, to the general employment of a recognised system of 'accidental' sounds[3]; and, in process of time, to the unrestricted use of the Æolian and Ionian Modes—the prototypes of our Major and Minor Scales. These changes naturally prepared the way for the unprepared Dissonances of Monteverde; and, with the introduction of these, the old system was suddenly brought to an end, and our present Tonality firmly established upon its ruins.
Our present Major Scale is formed of two Tetrachords, separated by a greater Tone: the Semitone, in each, occurring between the two highest sounds.
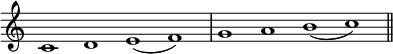
Our Minor Scale is formed of two dissimilar Tetrachords, also disjunct (i.e. separated by a greater Tone); in the uppermost of which the Semitone occurs between the two gravest sounds, as at (a); while, in the lower one, it is placed between the two middle ones; as at (b) (b).

This last Tetrachord maintains its form unchanged, whether the Scale ascend or descend; but, in the ascending Minor Scale, the upper Tetrachord usually takes the form of those employed in the Major Mode.

[ W. S. R. ]
- ↑ A description of the Greek Tetrachords would be quite beside the purpose of the present article. Those who wish for a closer acquaintance with the peculiarities of the Greek Scale will do well to consult a little tract, by General Perronet Thompson, called 'Just Intonation' (London, Effingham Wilson, 11 Royal Exchange).
- ↑ See Hexachord.
- ↑ See Musica Ficta.
