An introduction to linear drawing/Chapter 1
PART FIRST.
FIRST CLASS.


The first class only draw right lines, triangles and perpendiculars. The corrections are made with a rule, and dividers.
The four first figures drawn above, relate particularly to the first eight propositions. To ascertain if the line be straight, let the monitor draw a line through it or near it with a rule. To ascertain if a line be cut into equal parts, measure the parts with the dividers, if the eye be not sufficiently practised to detect the errors without their assistance.
PROPOSITIONS.
1. Draw a right line (that is, a straight line.) fig. 1.
2. Draw a right line and divide it in the centre. fig. 2.
3. Draw a right line and cut it into quarters. fig. 3.
4. Draw a right line and lengthen it a much farther. fig. 2.
5. Draw a right line and continue it twice length. (fig. 4.)
6. Draw a right line and lengthen it three times its length. (fig. 3)
7. Cut a right line into three equal parts. (fig 4.)
8. Cut a right line into six or eight equal parts, and so on.
It will be a useful exercise at this stage of the business, to show the parts of a line when divided. Thus, if required to show how much three quarters of a line are, the pupil must find one quarter, and the rest of the line will be three quarters. To find three fifths of line, cut the line into five parts, and take three of them. A very correct idea of fractions may in this way be communicated.

9. Draw a line one inch long, then two, three, four, five, six. (fig. 5.)
10. Draw a line and divide it into inches.
It is of no consequence what the length of the line is. Begin at the left and mark as many whole inches as there may be.
A horizontal line is one drawn from left to right, or from right to left. The surface or top of a bowl of water is horizontal or level.
12. Draw a perpendicular line. (fig. 6.)
A perpendicular or vertical line is one perfectly upright, as a string, with a weight at the end of it, will hang from a nail, or from the hand.
In making horizontal lines, the pupil should make them parallel to the top or bottom of his slate or paper, and in making perpendiculars they should be parallel to the sides of the slate or paper. Parallel lines are lines running in the same direction equally distant from each other in every part; thus, the horizontal lines in figures 1, 2, 3, 4, are parallel to each other. Lines may be drawn parallel at any distance from each other.
18. Draw two parallel horizontal lines, then three, four, five and six.
14. Draw two parallel perpendiculars, then three, four, five and six.
15. Draw an oblique line and cut it into two, four, three and six parts.
An oblique line is one between a horizontal and a perpendicular; that is, a leaning line.
16. Draw two parallel obliques, then three, four, five and six.
17. Draw parallel lines an inch apart, then half an inch, a quarter, &c.
18. Draw a perpendicular, and cut it into two, three four, five, six equal parts.
It is difficult to cut a perpendicular into equal parts, because of an optical deception which leads us to think the upper parts shorter than they really are. This must be guarded against.
19. Join two dots or points by a right line.
The pupil will move his pencil two or three times from the left dot to the right, before he draws the line. This precaution is more necessary when the operation is performed on paper than when on a slate, where it may be erased if wrong.

20. Make an acute angle. (fig. 7.)
Care must be taken to distinguish an angle, from what is called its point or apex. The angle is the opening between two lines that meet, and the point or apex is the point where the lines meet. A pair of dividers forms a number of different angles, by being opened more or less.
It is this opening of the sides which determines the size of the angle, and not the length of the sides, which, if lengthened out ever so far, would not affect the size of the angle, because the opening will only be the same part of a a great circle that it was of a small one.

Imagine two lines which cross each other as in figures 9 and 10. They will make four angles. These are right angles if they are equal, and they will be equal if one line is perpendicular to the line it crosses. If the angle be less than a right angle, it is called an acute angle; if more, it is called an obtuse angle. Acute means sharp, and obtuse means blunt.
21. Make an obtuse angle. (fig. 8.)
22. Make an acute angle with the opening turned upward, downward, to the right and to the left.
23. Make a triangle. (fig. 11.)

Close the space between the sides of an angle with a right line, and you make a triangle, a figure which has three angles and three sides.
The base is the side on which the triangle is supposed to rest.
The apex of a triangle is the point opposite to the base.
The height of a triangle is a perpendicular drawn from the apex to the base. In the figure it is shown by the dotted line.
A triangle is called Isoceles when two sides are equal. If all three of the sides are equal, it is Equilateral, (which word means equal-sided;) and if all the sides are unequal, it is called Scalene.
24. Raise a perpendicular on a horizontal. (fig. 9.)
This will produce right angles, as we have before remarked. To ascertain if the angle be exact, take a piece of what is called bonnet paper or thin pasteboard, cut it round and then cut the round piece into quarters. Each quarter will have two sides at right angles, and by inserting the apex into the opening of the angle drawn by the pupil, any incorrectness will be detected. A small brass or iron square will serve the same purpose, but does not satisfactorily show that a right angle is equal to a quarter of a circle, which is also called a quadrant.
25. Cross a right line with a perpendicular. (fig. 10.)
The right line should be drawn in various directions, to show the pupil that a perpendicular may be raised on any right line, whether horizontal or oblique.
26. Draw a rectangular, or right angled triangle, (figs. 12 and 13.)
This is a triangle of which one of the angles is a right angle, as the lower left hand one in fig. 12, and the top one in fig. 13. The base may be horizontal or inclined.
27. Make a rectangular isoceles triangle.
There is no difference between this and figures 12 and 13, except that in an isoceles triangle, two of the sides must be of equal length. In fact, fig. 12 is an isoceles.

Figure 13, though rectangular, is a scalene also.
28. Draw a rectangle. (fig. 14.)
A rectangle is properly a figure with four sides, of which each two opposite sides are equal and parallel, and of which all the angles are right angles.

The lower side is the base, and the right or left side is the height.
To ascertain its correctness, the Monitor may examine every angle with his quadrant of pasteboard, or he may with his dividers see if the left hand upper, and right hand lower angles are as far apart as the other two angles are. Figure 14 is what is often called a long or oblong square.
29.Make a rectangle, and cut it into equal right angles. fig. 15.
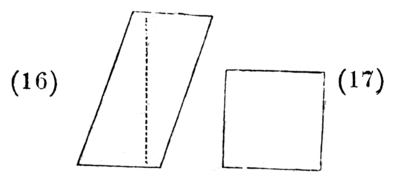
30. Make a parallelogram, and mark its height. (fig. 16.)
The parallelogram, like the rectangle, has its opposite sides parallel, but none of its angles are right. The height is a perpendicular dropped from the top to the base, and is marked by the dotted line in the figure.
31. Make a square. (fig. 17.) This figure has all its sides equal, and all its angles right.

32. Draw two angles with parallel sides. (figs. 18 and 19.)
Two angles, as in figure 18, are called parallel, not because their sides are of equal length, but because their openings and points correspond exactly. Fig. 19 is designed to exercise the pupil in making parallel angles in various positions.
33.Draw obliques equidistant (that is, equally distant) from a perpendicular.
Draw first a horizontal, raise a perpendicular on its centre, and then draw a line from the top of the perpendicular to each end of the horizontal. The figure will then be an isoceles triangle, as in fig. 11.
34.Make a scalene triangle.(fig. 13.)
As it is not difficult to make a triangle of unequal sides, it will be well for the monitor to prescribe the length of one or more of them. Thus he may say: "Make a scalene triangle, of which the three sides shall measure one inch and two tenths; one inch; and eight tenths of an inch."
35.Make an equilateral triangle.(fig. 20.)
After the pupil makes the figures exactly, let the length of the sides he given, as one, two, three, &c. inches. Then require the point to be under the base, turned to the right, &c.
36.From a given point draw a perpendicular.
First draw a right line, then make the proposed point, and lastly draw the perpendicular.
27.Raise a perpendicular on the end of a right line.

38.Make a Rhomb or Lozenge.(fig. 21.)
The four sides are equal as in the square, but the angles are not right angles. To draw this figure, make a right line, cross it with a perpendicular, like the dotted lines in the figure, and then draw the sides.
If the Rhomb or Lozenge have all the angles equal, the figure is merely a square placed obliquely, as in fig. 22.

39.Cut a rectangle into halves.(fig. 23.)
This will make two angles, whose exactness may be tested by an eighth part of a circle of pasteboard, the rectangle being quarter of a circle, as was stated under Prop. 24.
40.Cut an acute angle into two equal parts.(fig.24.)
41.Double an angle.
Make an angle of any size, and then make another of the same size by the side of it. Suppose the lower angle of fig. 24 to be made first, then by making the upper right line, the angle will be doubled.

42.Triple an angle.(fig. 25.)
43.Cut an angle into three equal parts.(fig. 25.)
44.Cut an angle into six equal parts.(fig. 26.)
These three propositions need no explanation.
