Elements of the Differential and Integral Calculus/Chapter X

by William Anthony Granville

CHAPTER X

RATES

94. The derivative considered as the ratio of two rates. Let

$y=f(x)$

be the equation of a curve generated by a moving point P. Its coördinates x and y may then be considered as functions of the time, as explained in § 71. Differentiating with respect to t, by XXV, §33, we have

Plot of curve generated by P.

(32)	${\frac {dy}{dt}}=f'(x){\frac {dx}{dt}}.$

At any instant the time rate of change of y (or the function) equals its derivative multiplied by the time rate change of the independent variable.

Or, write (32) in the form

(33)	${\frac {\frac {dy}{dt}}{\frac {dx}{dt}}}=f'(x)={\frac {dy}{dx}}.$

The derivative measures the ratio of the time rate of change of y to that of x.

${\tfrac {ds}{dt}}$ being the time rate of change of length of arc, we have from (12),§71,

(34)	${\frac {ds}{dt}}={\sqrt {\left({\frac {dx}{dt}}\right)^{2}+\left({\frac {dt}{dt}}\right)^{2}}}.$

which is the relation indicated by the above figure.

As a guide in solving rate problems use the following rule:

First Step. Draw a figure illustrating the problem. Denote by x, y, z, etc., the quantities which vary with the time.

Second Step. Obtain a relation between the variables involved which will hold true at any instant.

Third Step. Differentiate with respect to the time.

Fourth Step. Make a list of the given and required quantities.

Fifth Step. Substitute the known quantities in the result found by differentiating (third step), and solve for the unknown.

EXAMPLES

1. A man is walking at the rate of 5 miles per hour towards the foot of a tower 60 ft. high. At what rate is he approaching the top when he is 80 ft. from the foot of the tower?

Example 2 illustration.

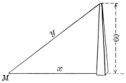
Solution. Apply the above rule.
First step. Draw the figure. Let x = distance of the man from the foot and y = his distance from the top of the tower at any instant.
Second step. Since we have a right triangle,
$\ y^{2}$	$=x^{2}+3600\$.
Third step. Differentiating, we get
$2y{\frac {dy}{dt}}$	$=2x{\frac {dx}{dt}}$, or,
(A) ${\frac {dy}{dt}}$	$={\frac {x}{y}}{\frac {dx}{dt}}$, meaning that at any instant whatever
(Rate of change of y)	= $\left({\frac {x}{y}}\right)$ (rate of change of x).

Fourth step.	$\ x$	$=80,\$	${\frac {dx}{dt}}$	$=5\$ miles an hour,
				= 5 × 5280 ft. an hour.
	$y$	$={\sqrt {x^{2}+3600}}$	${\frac {dy}{dt}}$	= ?
		= 100.
Fifth step.	Substituting back in (A),
	${\frac {dy}{dt}}$	= ${\frac {80}{100}}\times 5\times 5280$ ft. per hour
		= 4 miles per hour. Ans.

2. A point moves on the parabola $6y=x^{2}$ in such a way that when x = 6, the abscissa is increasing at the rate of 2 ft. per second. At what rates are the ordinate and length of arc increasing at the same instant?

Solution First step. Plot the parabola.
Second step.	$\ 6y$	$=x^{2}\$
Third step.	$6{\frac {dy}{dt}}$	$=2x{\frac {dx}{dt}}$, or,
(B)	${\frac {dy}{dt}}$	$={\frac {x}{3}}\cdot {\frac {dx}{dt}}$.
This means that at any point on the parabola
(Rate of change of ordinate)		= $\left({\frac {x}{3}}\right)$(rate of change of abcissa).

Fourth step.	${\frac {dx}{dt}}$	= 2 ft. per second
	$x=6\$.	${\frac {dy}{dt}}$	= ?
	$y={\frac {x^{2}}{6}}=6$.	${\frac {ds}{dt}}$	= ?
Fifth step. Substituting back in (B),
	${\frac {dy}{dt}}={\frac {6}{3}}\times 2=4$ ft. per second. Ans.

From the first result we note that at the point P(6, 6) the ordinate changes twice as rapidly as the abscissa.

If we consider the point P'(-6, 6) instead, the result is ${\tfrac {dy}{dt}}=-4$ ft. per second, the minus sign indicating that the ordinate is decreasing as the abscissa increases.

3. A circular plate of metal expands by heat so that its radius increases uniformly at the rate of .01 inch per second At. what rate is the surface increasing when the radius is two inches?

Circular plate of metal.

Solution. Let x = radius and y = area of plate. Then
	$\ y$	$=\pi x^{2}\$.
(C)	${\frac {dy}{dt}}$	$=2\pi x{\frac {dx}{dt}}$,

That is; at any instant the area of the plate is increasing in square inches $2\pi x$ times as fast as the radius is increasing in linear inches.

	$\ x$	$=2,{\frac {dx}{dt}}=.01,{\frac {dy}{dt}}=?$
Substituting in (C),
	${\frac {dy}{dt}}$	$=2\pi \times 2\times .01=.04\pi$ sq. in. per sec. Ans.

4. An arc light is hung 12 ft. directly above a straight horizontal walk on which a boy 5 ft. in height is walking. How fast is the boy's shadow lengthening when he is walking away from the light at the rate of 168 ft. per minute?

Arc light over a straight walk.

Solution. Let x = distance of boy from a point directly under light L, and y = length of boy's shadow. From the figure,
	$y:y+x::5:12\$,
or	$\ y$	$={\frac {5}{7}}x$.
Differentiating,	${\frac {dy}{dt}}$	$={\frac {5}{7}}{\frac {dx}{dt}}$;

i.e. the shadow is lengthening ${\tfrac {5}{7}}$ as fast as the boy is walking, or 120 ft. per minute.

5. In a parabola $y^{2}=12x$, if x increases uniformly at the rate of 2 in. per second, at what rate is y increasing when x = 3 in. ? Ans. 2 in. per sec. 6. At what point on the parabola of the last example do the abscissa and ordinate increase at the same rate? Ans. (3,6).

7. In the function $y=2x^{3}+6$, what is the value of x at the point where y increases 24 times as fast as x? Ans. $x=\pm 2$.

8. The ordinate of a point describing the curve $x^{2}+y^{2}=25$ is decreasing at the rate of 1½ in. per second. How rapidly is the abscissa changing when the ordinate is 4 inches? Ans. ${\tfrac {dx}{dt}}$ = 2 in. per sec.

9. Find the values of x at the points where the rate of change of

$x^{3}-12x^{2}+45x-13$

is zero. Ans. x = 3 and 5.

10. At what point on the ellipse $16x^{2}+9y^{2}=400$ does y decrease at the same rate that x increases? Ans. (3, ${\tfrac {16}{3}}$).

11. Where in the first quadrant does the arc increase twice as fast as the ordinate? Ans. At 60°.

A point generates each of the following curves. Find the rate at which the arc is increasing in each case:

12. $y^{2}=2x;{\frac {dx}{dt}}=2,x=2$.	Ans.	${\frac {ds}{dt}}={\sqrt {5}}$.
13. $xy=6;{\frac {dy}{dt}}=2,y=3$.		${\frac {ds}{dt}}={\frac {2}{3}}{\sqrt {13}}$.
14. $x^{2}+4y^{2}=20;{\frac {dx}{dt}}=-1,y=1$.		${\frac {ds}{dt}}={\sqrt {2}}$.
15. $y=x^{3};{\frac {dx}{dt}}=3,x=-3$.
16. $y^{2}=x^{3};{\frac {dy}{dt}}=4,y=8$.

17. The side of an equilateral triangle is 24 inches long, and is increasing at the rate of 3 inches per hour. How fast is the area increasing? Ans. $36{\sqrt {3}}$ sq. in. per hour.

18. Find the rate of change of the area of a square when the side b is increasing at the rate of a units per second. Ans. 2 ab sq. units per sec.

19. (a) The,volume of a spherical soap bubble increases how many times as fast as the radius? (b) When its radius is 4 in. and increasing at the rate of ½ in. per second, how fast is the volume increasing? Ans. (a) 4πr² times as fast; (b) 32π cu. in. per sec.

How fast is the surface increasing in the last case?

20. One end of a ladder 50 ft. long is leaning against a perpendicular wall standing on a horizontal plane. Supposing the foot of the ladder to be pulled away from the wall at the rate of 3 ft. per minute; (a) how fast is the top of the ladder descending when the foot is 14 ft. from the wall? (b) when will the top and bottom of the ladder move at the same rate? (c) when is the top of the ladder descending at the rate of 4 ft. per minute? Ans. (a) ${\tfrac {7}{78}}$ ft. per min.; (b) when $25{\sqrt {2}}$ ft. from wall; (c) when 40 ft. from wall.

21. A barge whose deck is 12 ft. below the level of a dock is drawn up to it by means of a cable attached to a ring in the floor of the dock, the cable being hauled in by a windlass on deck at the rate of 8 ft. per minute. How fast is the barge moving towards the dock when 16 ft. away? Ans. 10 ft. per minute. 22. An elevated car is 40 ft. immediately above a surface car, their tracks intersecting at right angles. If the speed of the elevated car is 16 miles per hour and of the surface car 8 miles per hour, at what rate are the cars separating 5 minutes after they meet? Ans. 17.9 miles per hour.

23. One ship was sailing south at the rate of 6 miles per hour; another east at the rate of 8 miles per hour. At 4 P.M. the second crossed the track of the first where the first was two hours before; (a) how was the distance between the ships changing at 3 P.M.? (b) how at 5 P.M.? (c) when was the distance between them not changing? Ans. (a) Diminishing 2.8 miles per hour; (b) increasing 8.73 miles per hour; (c) 3: 17 P.M.

24. Assuming the volume of the wood in a tree to be proportional to the cube of its diameter, and that the latter increases uniformly year by year when growing, show that the rate of growth when the diameter is 3 ft. is 36 times as great as when the diameter is 6 inches.

25. A railroad train is running 15 miles an hour past a station 800 ft. long, the track having the form of the parabola

$y^{2}=600x,$,

and situated as shown in the figure. If the sun is just rising in the east, find how fast the shadow S of the locomotive L is moving along the wall of the station at the instant it reaches the end of the wall.

Track with shape of a parabola.

Solution.	$y^{2}$	$=600x$
	$2y{\frac {dy}{dt}}$	$=600{\frac {dx}{dt}}$,
or	${\frac {dx}{dt}}$	$={\frac {y}{300}}{\frac {dy}{dt}}$.

Substituting this value of ${\frac {dx}{dt}}$ in

	${\frac {ds}{dt}}$	$={\sqrt {\left({\frac {dx}{dt}}\right)^{2}+\left({\frac {dy}{dt}}\right)^{2}}}$, we get
(D)	$\left({\frac {ds}{dt}}\right)^{2}$	$=\left({\frac {y}{300}}{\frac {dy}{dt}}\right)^{2}+\left({\frac {dy}{dt}}\right)^{2}$.
Now	${\frac {ds}{dt}}$	= 15 miles per hour dt
		= 22 ft. per sec.
	$y$	= 400 and ${\frac {dy}{dt}}$ = ?

Substituting back in (D), we get

	$\ (22)^{2}$	$=\left({\frac {16}{9}}+1\right)\left({\frac {dy}{dt}}\right)^{2}$,
or,	${\frac {dy}{dt}}$	= $13{\frac {1}{5}}$ ft. per second. Ans.

26. An express train and a balloon start from the same point at the same instant. The former travels 50 miles an hour and the latter rises at the rate of 10 miles an hour. How fast are they separating? Ans. 51 miles an hour. 27. A man 6 ft. tall walks away from a lamp-post 10 ft. high at the rate of 4 miles an hour. How fast does the shadow of his head move? Ans. 10 miles an hour.

28. The rays of the sun make an angle of 30° with the horizon. A ball is thrown vertically upward to a height of 64 ft. How fast is the shadow of the ball moving along the ground just before it strikes the ground? Ans. 110.8 ft. per sec.

29. A ship is anchored in 18 ft. of water. The cable passes over a sheave on the bow 6 ft. above the surface of the water. If the cable is taken in at the rate of 1 ft. a second, how fast is the ship moving when there are 30 ft. of cable out? Ans. $1{\frac {2}{3}}$ ft. per sec.

30. A man is hoisting a chest to a window 50 ft. up by means of a block and tackle. If he pulls in the rope at the rate of 10 ft. a minute while walking away from the building at the rate of 5 ft. a minute, how fast is the chest rising at the end of the second minute? Ans. 10.98 ft. per min.

31. Water flows from a faucet into a hemispherical basin of diameter 14 inches at the rate of 2 cu. in. per second. How fast is the water rising (a) when the water is halfway to the top? (b) just as it runs over? (The volume of a spherical segment = ${\tfrac {1}{2}}\pi r^{2}h+{\tfrac {1}{6}}\pi h^{3}$, where h = altitude of segment.)

32. Sand is being poured on the ground from the orifice of an elevated pipe, and forms a pile which has always the shape of a right circular cone whose height is equal to the radius of the base. If sand is falling at the rate of 6 cu. ft. per sec., how fast is the height of the pile increasing when the height is 5 ft.?

33. An aeroplane is 528 ft. directly above an automobile and starts east at the rate of 20 miles an hour at the same instant the automobile starts east at the rate of 40 miles an hour. How fast are they separating?

34. A revolving light sending out a bundle of parallel rays is at a distance of t a mile from the shore and makes 1 revolution a minute. Find how fast the light is traveling along the straight beach when at a distance of 1 mile from the nearest point of the shore. Ans. 15.7 miles per min.

35. A kite is 150 ft. high and 200 ft. of string are out. If the kite starts drifting away horizontally at the rate of 4 miles an hour, how fast is the string being paid out at the start? Ans. 2.64 miles an hour.

36. A solution is poured into a conical filter of base radius 6 cm. and height 24 cm. at the rate of 2 cu. cm. a second, and filters out at the rate of 1 cu. cm. a second. How fast is the level of the solution rising when (a) one third of the way up? (b) at the top? Ans. (a) .079 cm. per sec.; (b) .009 cm. per sec.

37. A horse runs 10 miles per hour on a circular track in the center of which is an arc light. How fast will his shadow move along a straight board fence (tangent to the track at the starting point) when he has completed one eighth of the circuit? Ans. 20 miles per hour.

38. The edges of a cube are 24 inches and are increasing at the rate of .02 in. per minute. At what rate is (a) the volume increasing? (b) the area increasing?

39. The edges of a regular tetrahedron are 10 inches and are increasing at the rate of .3 in. per hour. At what rate is (a) the volume increasing? (b) the area increasing?

40. An electric light hangs 40 ft. from a stone wall. A man is walking 12 ft. per second on a straight path 10 ft. from the light and perpendicular to the wall. How fast is the man's shadow moving when he is 30 ft. from the wall? Ans. 48 ft. per sec.

Drawbridge.

41. The approach to a drawbridge has a gate whose two arms rotate about the same axis as shown in the figure. The arm over the driveway is 4 yards long and the arm over the footwalk is 3 yards long. Both arms rotate at the rate of 5 radians per minute. At what rate is the distance between the extremities of the arms changing when they make an angle of 45° with the horizontal? Ans. 24 yd. per min.

42. A conical funnel of radius 3 inches and of the same depth is filled with a solution which filters at the rate of 1 cu. in. per minute. How fast is the surface falling when it is 1 inch from the top of the funnel? Ans. ${\frac {1}{4\pi }}$ in. per mm.

43. An angle is increasing at a constant rate. Show that the tangent and sine are increasing at the same rate when the angle is zero, and that the tangent increases eight times as fast as the sine when the angle is 60°.

---

---