Great Neapolitan Earthquake of 1857/Part II. Ch. X

by Robert Mallet

CHAPTER X.

PADULA AND ITS NEIGHBOURHOOD—THE PALAZZO ROMANI AND ITS GARDENS.

---

Padula, a large town, said to be of Lucanian origin, but with no traces of extreme antiquity, and generally well built, stands upon a pretty steep eminence of solid limestone, at the southern extremity of the first great break in the lateral chain to the east of the Vallone. The range rises high above to the N. N. E.; and eastward of the same there is a narrow lateral valley in a direction about N. E., and exactly at the tongue, between it and the great Vallone stands Padula. The Vallone here narrows very rapidly, and trends off to the westward at about a mile or two south; and still further south at Buona Bitacola on the west, and Montesano on the east, it becomes quite hemmed in by lofty mountains. Violent contrary motions must therefore have been produced by the breaking up of the great wave of shock in the deep alluvium, and its in-baying and shelving up upon the irregular masses of limestone mountains that divide the many divergent valleys, and gorges, and project between them. Directly south of Padula, and of the great Cistercian Monastery of St. Lorenzo, about a mile west of the town, the clays of the piano give place to a rolling and broken surface of clay and gravel, overlying limestone rock at a greater or less depth, and extending for more than four square miles; above which to the east rise the summits of Monte St. Elia, and Monte della Vajana, which recommence the broken chain of the E. lateral range. Padula therefore, has got, soft, cretaceous, and sandy, white limestone beds with east and west strike, and nearly vertical stratification to the N., similar but harder limestone of unknown stratification to the south, and the deep clays of the piano to the west of it. The rock upon which the town stands is of the same quality and stratification as the range to the north, and the colline is joined on to the great range by a shoulder at rather a lower level. The western and southern slopes of the town are very steep, averaging probably 30° from the horizon towards the plain; the buildings generally, either founded on the bare rock, or upon a thin stratum of diluvial matter, which increases in depth as we descend towards the plain.

The buildings on these sides are the oldest and worst, and have suffered the most, having been exposed to the severest brunt of the shock, and been the least able to bear it, and in the most favourable position heaped above each other to produce mutual destruction in falling. The counterscarp of the town, or that on the east and N. E. towards the narrow valley and gorge behind it, is less steep close to the town, but within a quarter of a mile of both sides of the gorge becomes precipitous; the west side being bare limestone rock nearly to the bottom, which is not much above the level of the piano (at the town), and the opposite, or east side, covered with steep banks of calcareous clays; limestone, angular gravel, and sand, getting very deep and heavy as they descend to the bottom, where they are being cut into and carried away by the torrent and rivers round the town to the east and south, and passing the Certosa de S. Lorenzo farther into the Calore. This torrent issues principally from copious springs in the rock and coming from under the clays, about a mile and a half up the gorge to the north; and these are now, and have been ever since the earthquake, highly turbid and discoloured by the reddish earth, and are said by the Syndic and Subjudice of Padula, who visited the place with me, to be largely increased in volume of water, since that event.

The Photog. No. 210, is taken in this valley, looking back at the town toward the S. W. On the steep counterscarp to the right of this view I found a large fissure in the solid clay covering, extending some 300 yards in length at about 270 feet above the bottom of the gorge. The direction was due E. and W. by compass, and its S. slip was from 1 to 7 inches below the level of the opposite one. The fissure is a flowing curve similar in horizontal plan to the contour of the hill side, and its line of direction is just that most favourable, to a throw off and slip of the clay masses, upon the sublying rock, by the jog of the earthquake, which is unquestionably the nature of its formation. Upon the opposite side, and about half a mile up the valley to the north, where the east slope has become much steeper, I observe with the telescope that huge masses of clay and gravel that had stood above the torrent as nearly vertical hanks, of from 50 to 120 feet in height over the water, have in several places been shaken down, and fallen in great masses, damming the torrent into large and very deep, discoloured pools, from some of which the dams have already given way by little debacles, while in several of the others, the water is escaping beneath and through the clay, and carrying volumes of fluid mud and sand away with it: a very few weeks with heavy rain, will carry tens of thousands of tons of clay and gravel down into the Calore.

Higher up the gorge than the Photog. view extends, and upon the east flank of the gorge, this slope becomes very precipitous, and consists of bare cretaceous limestone, standing up in aiguilles and isolated weathered masses, some of great magnitude, and here (as well as from some points on the rocky counterscarps of the town colline), ponderous falls of rock have taken place. The massive fragments with newly-broken and glittering white surfaces encumbering the slope, or, after having traced their descending paths in lines of torn rock and furrowed detritus, block up the bed of the torrent below, which brawls between the immense fragments and beneath them. Most of them have been detached from the outcropped ends of the ill-discernible vertical strata, separating at joints, &c., and are mere cases of loss of equilibrium by the shock; but one most remarkable case I observed, in which an enormous mass of solid rock that had stood up as a sort of blunt aiguille from the steep face of the slope, not quite vertical, but rather overhanging, (about 15° by the eye,) to the downward side, had been broken clean off at its base, and again breaking into three massive pieces, had slid down the rocky slope, and now occupied a place about 150 feet below, having crossed and wholly torn away the mule-path in their progress. The appearance of these masses, as they must have been prior to their fall, may be gathered from Sketch No. 211, and the way in which the fragments lay from Sketch No. 212.

The aiguille, by measurements of the three principal fragments, must have stood about 70 feet high above its base when entire, and the three masses, when roughly cubed, contained fully 35,000 cubic feet, and weighed about 2,500 tons. The surface of fracture, of which about 2/5ths was formed of planes of separation (transverse joints) in the rock, and the remainder through the solid stone, was an irregular circular figure, and about 20 ft. × 18 ft., the plane of fracture generally being inclined about 45° to the horizon.

The rock was very soft, arenaceous, and chalky limestone, perfectly white, and a hand specimen, when fresh fractured, could, like sugar, be rubbed away against another piece; so that the cohesive energy was not great, and the inertia of so great and high a mass was quite sufficient, at a very moderate velocity, to bring it over. It however has a peculiar interest, as the first example I have seen of actual rock fracture by the direct operation of the shock.

Neither the position of the centre of gravity, nor of that of resistance of the fractured base could be fixed with sufficient accuracy to enable any calculation to be based upon the fracture and fall of the mass, that would give a trustworthy measure of velocity or of direction.

On examining the buildings in the town on the south and south-west slopes, where the damage done was greatest, I found evidence by fissures and projected wedges of masonry, giving a direction of wave-path varying between the limits of 155° E. of north to 167° E. of north, and angles of emergence (all, however, proving emergence from the north and N.W.) varying between 20° and 25°. Some of the fractures from which the latter elements are taken are visible in the Photog. No. 210, though not so in its lithographic reproduction.

Many circumstances indicated the transit of two shocks crossing obliquely, such as the twisting of objects on their bases, and the almost universal occurrence of fissures in all four walls of rectangular buildings, whether cardinal or ordinal.

At the very top of the town, founded upon the bare limestone rock, which here protrudes to the surface everywhere, I observed an old and partly ruinous mansion, the Palazzo Romani, which, as well as its deserted gardens, afforded me some valuable data.

The palazzo is a large, nearly square, three-story building, very nearly cardinal. The walls are well built of rubble. It stands on level ground. It is fissured in all four external walls: all the fissures are long and threadlike, and are scarcely visible in the Photogs. No. 213 and No. 214 (Coll. Roy. Soc.), the former showing the S.W. quoin, the latter the opposite, or N.E. one. From previous indications of double shock here, I dare not conclude anything as to horizontal component of wave-path, from the fissures in adjacent walls; but those in the flank walls gave excellent evidence as to angle of emergence, which proved to be 25° to 25° 30' from north. The fissures in the adjacent walls were much more nearly vertical (8° to 10° inclination), and hence appeared to have been produced by the secondary shock, which shook the whole colline of the town to its base, and therefore by an oscillation more nearly in an horizontal path. I could only gain access to part of the interior; it was empty and disused, and offered little to record, probably.

In front, and to the N.W. of the palazzo, is a little columnar monument—Il Croce Romani, of the ordinary character; a Roman Doric column of 9 inches diameter of shaft and 9 feet high, supporting a white marble ball and cross, and placed upon a square pedestal and plinth block, elevated on a few courses of rude masonry as in Photog. No. 215 (Coll. Roy. Soc.), and Figs. 1, 2, and 3, Diagram No. 216. The ball and cross are secured to the top of the column, only by an iron dowal, and are loose; the plane of

the cross has been long twisted out of square, I was informed.

No injury has been sustained by this little structure, except that the pedestal has been thrown over, being lifted slightly at the south side by 1/4 of an inch vertically, as in Fig. 2, and jammed quite close to the plinth block at the opposite or north side. The shaft, pedestal, and plinth are socketed into each other, as shown, without cement.

A vertical plane passing through the axis of the column, &c., in a northerly and southerly direction ranges 25° E. of north. The lower edge of the pedestal is lifted more at the western than at the eastern corner of the south side, so as to infer a wave-path about 10° or 12° E. of north.

The shaft and pedestal, &c., are all of hard Apennine limestone, and the cavetto moulding above the base is 8 1/2 inches diameter only: an inference may be drawn from this as to the maximum possible velocity of shock, emergent at the angle we have found, viz., 25°, that would have left this little column unbroken at the neck formed by the cavetto. The horizontal velocity for fracture only is given by the Equation XXV.

$V={\tfrac {5}{8}}g\times {\frac {\mathrm {LD} }{a^{2}}}$;

where $\mathrm {D}$, the neck of the cavetto = 0.708 feet, $a$, the height of the column adding in the ball and iron, to the cylinder = 6.5 feet, and $\mathrm {L}$ = the length of the modulus of cohesion for the material. This will be = 225 feet, if we take the weight of a cubic foot = 160 lbs., and its cohesion at 500 lbs. per square inch (which is supported by Hodgkinson's experimental determination for marble, 551 lbs.). Then

$V=20.12\times {\frac {225\times .708}{42.25}}=75.961$ feet per second,

and

$V\sec e=75.961\times 1.103=\mathrm {V} =83.785$ feet per second;

the velocity at the emergence found, that would have just fractured the column at the cavetto.

It is, therefore, obvious that the velocity of the shock was greatly below that that would have been necessary to fracture the column by its own inertia of motion, even if the cohesion which I have assumed for the limestone of which it was formed be much above the truth, as is probably the case. The calculation, however, removes the wonder with which the unaided senses regard so slender a stalk of stone found whole and uninjured, in the midst of bowing roofs and massive walls rent or overthrown.

In what had once been the garden of the palazzo stood a portion of a shaft of an old column—one of six—that had once formed some sort of garden edifice. It had been broken off and overthrown, and afforded a precious admeasurement both for direction of wave-path and velocity of shock.

The columns had all been originally formed of an artificial "beton" or concrete, of broken limestone and brick, and lime mortar, rudely formed enough, without any base moulding but a fillet, and were 17 inches diameter. They stood upon the top course of a continuous base of limestone, running round a large circular raised platform of earth. They had all been broken down and the shafts had disappeared to within a few inches of their bases years since, except this one portion of a shaft, which stood before the earthquake 4 feet 8 inches above its base. For 6 inches in height the shaft was a united block, and fast to the stone base; at this height an old fracture had existed, and a portion of shaft, 2 feet 10 inches in length, had stood upon the lower block, having been replaced upon it, and a little fine mortar interposed in the old fracture. In toppling over, at the north side of the column, the arris was broken out, and the diameter in N. and S. direction reduced to 15 1/2 inches, as shown in Figs. 5 and 6, Diagram No. 221. Upon the top of this block, had stood another piece of the shaft 1 foot 4 inches high, cemented to it, in like manner at an old fracture. When examined by me, the two upper blocks had

been overthrown by the shock, and lay as in Figs. 5 and 6, Diagram 221, and as shown in Photog. No. 217, having never been moved or meddled with, the garden being an enclosure, since the earthquake. The lower and longer block was, at its lower end, within 9 inches of the stump of the shaft. The top block had been thrown over along with the one below it, had separated on striking the ground, at the top part, as the position of the other piece and the lower level of the soil than the stone base (Fig. 6) proved, and had slewed round and rolled a little off. The longer piece remained held by its impression in the soft soil precisely in the spot in which it had fallen.

The mortar joint at $c$, Fig. 6, had obviously possessed scarcely any bond whatever, and the only resistance to fall by the shock was, therefore, the stability of the superimposed two blocks upon the base or joint $c$. The form of the surface of separation, showed that the blocks had overturned precisely in a vertical plane, passing through the axis of the longer block as found prostrate, which corroborated the assurance of the Syndic and others present, that they had not been moved by any one since their fall. The direction of the axis of the fallen block, $\mathrm {B}$, was exactly 15° W. of N., and such was the wave-path that overthrew it, the movement having been from N. to S.

We may view the velocity that overthrew the shaft, separately from that which projected it from its base to the horizontal distance given, and compounding these two horizontal velocities, and resolving in the direction of the wave-path, obtain the total velocity impressed upon it, and hence that of the wave itself.

And first we obtain the horizontal velocity for overturning only from the equation

$V^{2}={\frac {15b^{2}+16a^{2}}{12a^{2}}}\times g{\sqrt {a^{2}+b^{2}}}(1-\cos {\theta })$

Here $a={\text{50 inches}},b={\text{17 inches}},\theta =19^{\circ }$; and solving for $V$ we have

$V^{2}={\frac {44335}{30000}}\times 2.683{\sqrt {2789}}(.0545)$

$=1.478\times 2.683\times 52.81\times .0545$

$V^{2}=11.42{\text{ and}}\therefore V={\text{3.38 feet per second,}}$

which is the horizontal velocity necessary to overturn the shaft only.

But it was also projected, so that a point taken at the lower arris of the overthrown shaft had moved horizontally a distance of 9 inches from the corresponding point of the base above $r$ (Fig. 6, Diagram No. 221), while the same point had descended vertically 6 inches from the arris of the base, as already described. Calling the horizontal ordinate A, and the vertical one B, we obtain the horizontal velocity of projection only from the equation

$V^{2}={\frac {g}{2}}\times {\frac {a^{2}}{b-a\tan {e}}}.$

Here $a={\text{0.75 feet, }}b={\text{0.50 feet, }}e=25^{\circ }\,30^{\prime }$

$V^{2}={\frac {32.2}{2}}\times {\frac {0.563}{0.500-0.750\times 0.4769}}$

$=16.1\times {\frac {0.563}{0.142}}$

$V^{2}=63.756{\text{ and }}V={\text{7.98 feet per second;}}$

adding the two horizontal velocities thus found, we obtain the total horizontal velocity impressed upon the shaft; or

$V=0.38+7.98={\text{11.36 feet per second.}}$

But this must be resolved to the direction of the wave-path, whose angle of emergence here we found to be

$e=25^{\circ }\,30^{\prime }.$

The velocity in this direction therefore is

$V\sec {e}=11.36\times \sec {e},$

or finally

$V=11.36\times 1.108={\text{12.586 feet per second,}}$

feet per second, which agrees very closely with previous determinations from other objects and at other stations.

The velocity thus obtained may be subject to two slight corrections, for which, however, the data are not obtainable. 1st. If the mortar at the old fracture of the base had any adhesion at all, (I believe it had none,) the velocity due to fracturing it, should be added.

2nd. The horizontal ordinate $a$ in the last equation is probably a little too great as measured, inasmuch as the fallen piece of the shaft must, by its own elasticity and that of the ground, have slid forward some fraction of an inch after it had struck the latter, which would make the velocity obtained a little too great.

These two errors, if they exist, tend to correct each other; and neither could affect the result to the extent of unity in the first decimal place. The result may therefore be relied upon.

In the same garden two vases of limestone, rudely hollowed out, had stood upon the opposite corners of the low parapet walls, that once confined the soil of beds, and separated them from broad walks between, now overgrown with dense turf and weeds, moss, twigs, &c.

These vases were both projected off their feet or lower portions, at a joint at $e$, Figs. 1 and 2, Diagram No. 222, and thrown to the ground, the lower portions remaining still upon the parapets, as seen in Photog. No. 220 (Coll. Roy. Soc.). One of the lower portions, ($\mathrm {A}$, Fig. 1,) I found square to the faces of the parapets, and obviously unmoved; the other, $\mathrm {B}$, had been twisted round 14°, so that two of its sides were in a line exactly north and south 14° W.

The lower portions at the joint $e$ (Figs. 1 and 3) were quite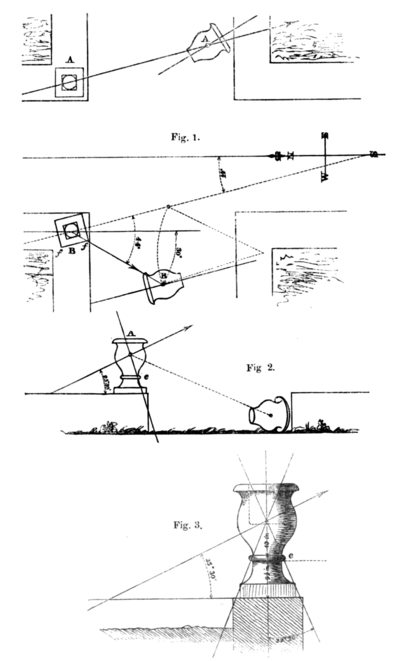

Diagram 222.

level and smooth, and the arrises perfect; and the upper and lower parts had been merely laid together, without any cementing material.

All the parts, like those of the column, &c. preceding, had remained untouched since their fall by the earthquake.

The centre of gravity of the upper part of each vase, (that projected off,) I found by trial was at 10 inches above the joint $e$, and the weight by trial, was 94 rotuli = 183 lbs. avoir. nearly.

The vase $\mathrm {A}$ had been thrown so, that its centre of gravity had been displaced 6.50 feet in the horizontal, and 3.60 feet in the vertical direction. We get the velocity of projection from the equation

$\mathrm {V} ^{2}={\frac {a^{2}g}{2\cos ^{2}e\left(b+a\tan e\right)}}$

in which $a=6.50$, $b=3.60$, $e=25^{\circ }30'$, as before.

Then

$\mathrm {V} ^{2}={\frac {42.25\times 32.2}{1.629\times \left(10.1\times 0.4769\right)}}={\frac {1360.50}{7.85}}$

$\mathrm {V} ^{2}=173\therefore V=13.152$ feet per second,

which only differs from the velocity given by the fallen column, by 0.566 foot, or a little more than 6 inches per second.

As respects horizontal direction, the vase A had been thrown in precisely the same path as the column preceding, viz., 15° W. of north towards the south; and its axis of symmetry was twisted as it lay, about 12° in a horizontal plane, from the vertical plane of projection, as seen in diagram, and it was obvious, from examination of the truf beneath, that it had not rolled after it struck the ground.

The vase $\mathrm {B}$, however, lay (as in Diagram) so, that its line of direction with its own base, was 30° E. of north, the horizontal range of throw being only=4.75 feet. There was evidence of this vase having rolled, from the point where it first alighted, westward, but the precise point at which it first struck the ground was not decisive. It appears probable that it was first, along with its base, tilted up upon the edge $ff$ by the transverse shock through the limestone, and that before it had returned to its former position, the principal or direct shock, in the direction 15° W. of north to south, caught it and threw the vase off the base; but part of the effort of projection was in that case destroyed by the fall back of vase and base, and hence the horizontal distance of projection is less than that of the other vase, and less than that due to the velocity of shock; and the direction of projection became one in some intermediate azimuth between the two wave-paths. We might assume that the vase, $\mathrm {B}$, was projected by one shock, and that, $\mathrm {A}$, by the other; in which case we could infer that the angle of horizontal intersection of the wave-paths had been = 45°, but that would render no account of the twisting of the base of $\mathrm {B}$. We must, therefore conclude, that the vase $\mathrm {B}$ was acted on by both shocks, and as it had rolled more or less, the angle of intersection would be less. The supposition made, also gives a solution for the fact otherwise hard to account for, that the part of this vase that rested on its base was towards the south as it lay, while the corresponding part of $\mathrm {A}$ faced the north. The latter was a clean throw, in the former the vase was tilted a little towards the N. E. in the return to its place, of its partly-upturned base, immediately previous to its throw, and the rotation thus commenced, turned it over in a vertical plane during its free descent from its base to the ground.

It will be seen, on looking at Fig. 3 (Diagram No. 222), that the vase might have been thus tilted upon its own base at the joint $e$, to the extent of nearly 22° 30′, before it would have lost the power of recovering its position, and that when tilted through part of this angle, the effect of the throw of the second shock delivered to it through the base, would tend to increase the rotation commenced, as well as to diminish the extent of horizontal range, or velocity impressed.

The general relation of all these objects as to azimuth, is shown in Fig. 223.

I found here also, as at Polla, an example of that singular circumstance, the keystone or block, of a cut stone semicircular arch over a doorway, which had worked up in place of coming down (by the movements of the earthquake) between the remaining arch stones. This case, of which the (original) Sketch No. 224 is a true representation, (though very imperfectly given by the woodcut,) is obviously due to the rocking to and fro of the whole wall in the plane of the arch, the motion being several times repeated, and hence the alternate partial freeing and gripping of the keystone, at $a$ and $c$, $b$ and $d$, between the rocking voussoirs, which, moving on the lowest point of the jambs, or at the springing level, are thus at each oscillation, relatively higher and lower than each other, at $d$ and at $a$. The keystone at each alternation, if already tolerably free above, by the fractures of the wall, produced at the first moment of shock is thus moved up more or less, and finally remains at the height gained.

In this instance, the keyblock had been thus lifted up, 1 1/4 inch above its former place. It is an excellent example, of one of the many cases, in which a misinterpretation of the phenomena, or misconception of the forces, &c., produces false notions as to the actual movement, of the shock productive of them. My attention was drawn to time arches, here and at Polla, by intelligent men (Syndici and Judici, &c.) as affording proof positive, of a sudden drop down, or jump up vertically, of the earth and the buildings upon it.