If and are the charges on a portion of the two cylinders of length , measured along the axis,
The capacity of a length of the interior cylinder is therefore
If the space between the cylinders is occupied by a dielectric of specific capacity instead of air, then the capacity of the inner cylinder is
The energy of the electrical distribution on the part of the infinite cylinder which we have considered is

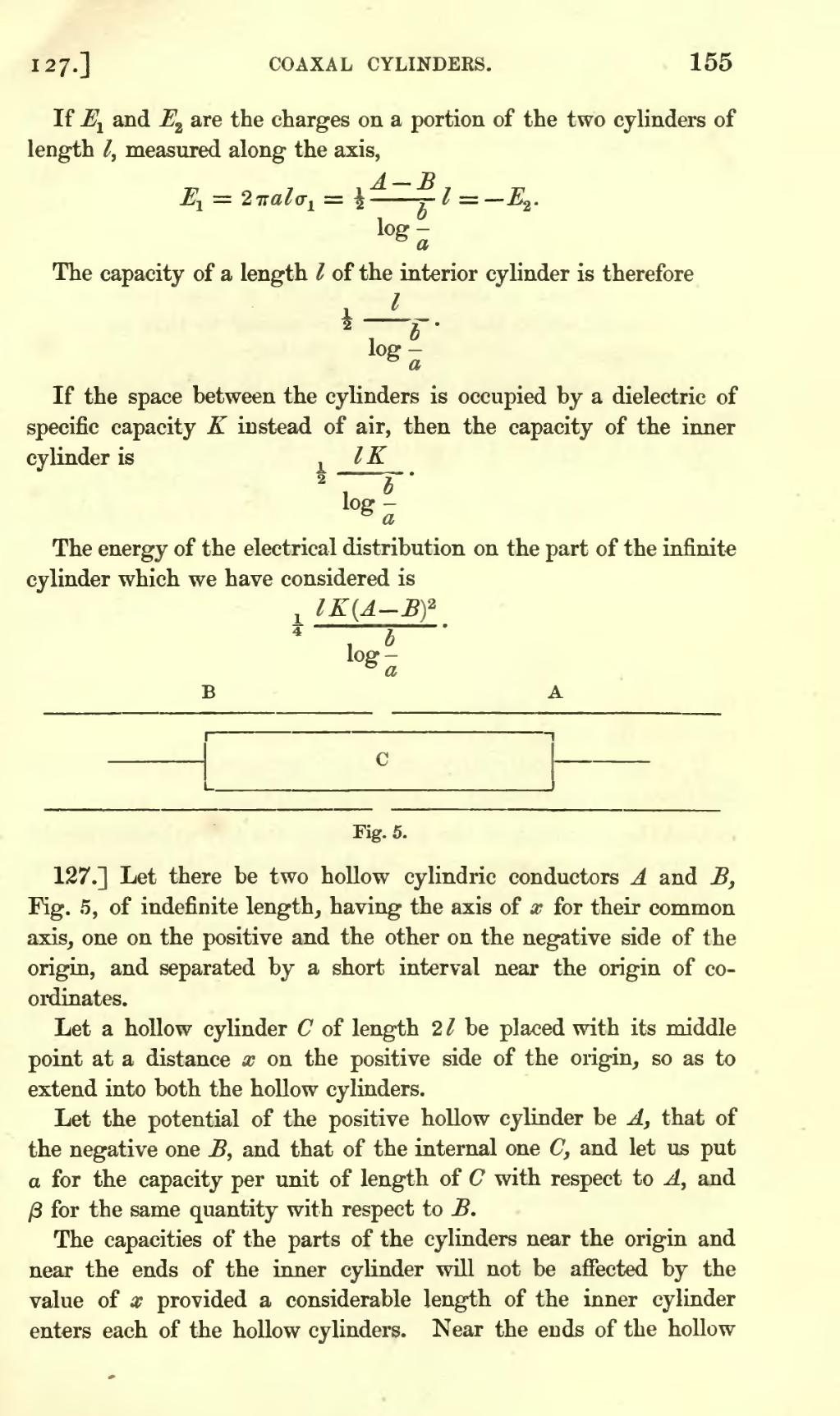
Fig. 5.
127.] Let there be two hollow cylindric conductors and , Fig. 5, of indefinite length, having the axis of for their common axis, one on the positive and the other on the negative side of the origin, and separated by a short interval near the origin of co ordinates.
Let a hollow cylinder of length be placed with its middle point at a distance on the positive side of the origin, so as to extend into both the hollow cylinders.
Let the potential of the positive hollow cylinder be , that of the negative one , and that of the internal one , and let us put for the capacity per unit of length of with respect to , and for the same quantity with respect to .
The capacities of the parts of the cylinders near the origin and near the ends of the inner cylinder will not be affected by the value of provided a considerable length of the inner cylinder enters each of the hollow cylinders. Near the ends of the hollow