Page:A Treatise on Electricity and Magnetism - Volume 1.djvu/231

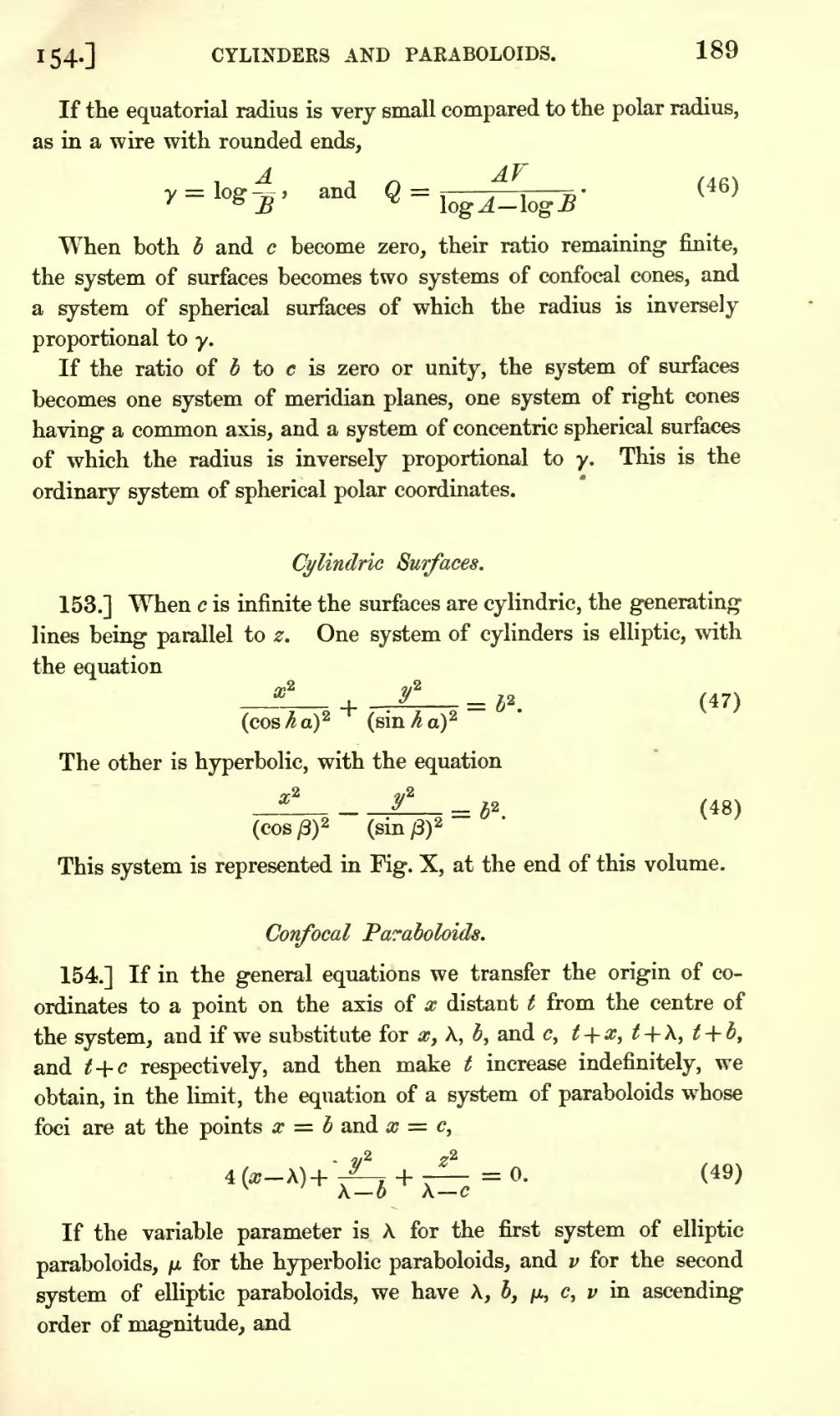
If the equatorial radius is very small compared to the polar radius, as in a wire with rounded ends,

$\gamma =\log {\frac {A}{B}},\ \mathrm {and} \ Q={\frac {AV}{\log A-\log B}}$

(46)

When both $b$ and $c$ become zero, their ratio remaining finite, the system of surfaces becomes two systems of confocal cones, and a system of spherical surfaces of which the radius is inversely proportional to $\gamma$.

If the ratio of $b$ to $c$ is zero or unity, the system of surfaces becomes one system of meridian planes, one system of right cones having a common axis, and a system of concentric spherical surfaces of which the radius is inversely proportional to $\gamma$. This is the ordinary system of spherical polar coordinates.

Cylindric Surfaces.

153.] When $c$ is infinite the surfaces are cylindric, the generating lines being parallel to $z$. One system of cylinders is elliptic, with the equation

${\frac {x^{2}}{(\cos h\alpha )^{2}}}+{\frac {y^{2}}{(\sin h\alpha )^{2}}}=b^{2}.$

(47)

The other is hyperbolic, with the equation

${\frac {x^{2}}{(\cos \beta )^{2}}}-{\frac {y^{2}}{(\sin \beta )^{2}}}=b^{2}.$

(48)

This system is represented in Fig. X, at the end of this volume.

Fig. X.

Confocal Paraboloids.

154.] If in the general equations we transfer the origin of co ordinates to a point on the axis of $x$ distant $t$ from the centre of the system, and if we substitute for $x,\lambda ,d,$ and $c,t+x,t+\lambda ,t+b$, and $t+c$ respectively, and then make $t$ increase indefinitely, we obtain, in the limit, the equation of a system of paraboloids whose foci are at the points $x=b$ and $x=c$,

$4(x-\lambda )+{\frac {y^{2}}{\lambda -b}}+{\frac {z^{2}}{\lambda -c}}=0.$

If the variable parameter is $\lambda$ for the first system of elliptic paraboloids, $\mu$ for the hyperbolic paraboloids, and $\nu$ for the second system of elliptic paraboloids, we have $\lambda ,b,\mu ,c,\nu$ in ascending order of magnitude, and