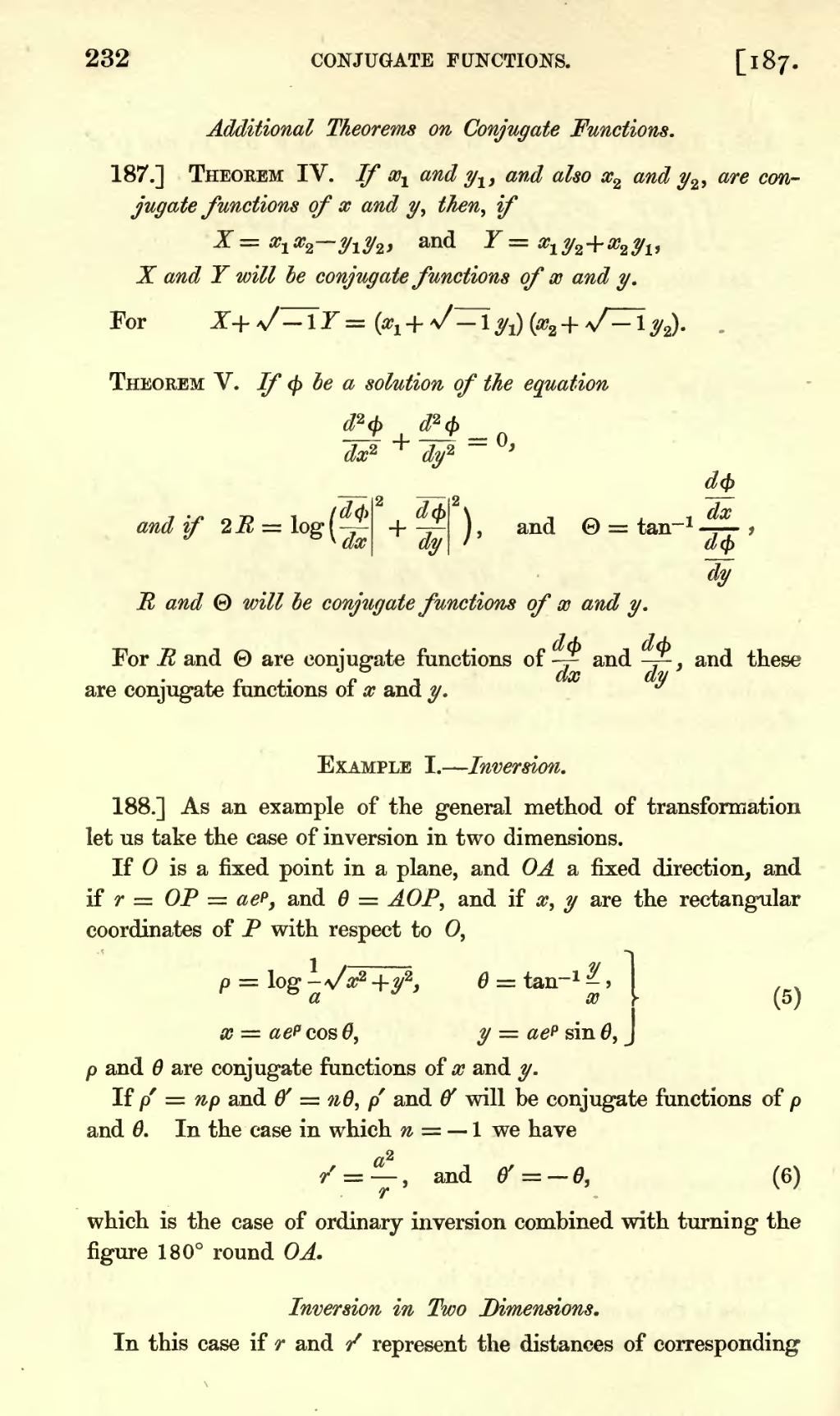
Additional Theorems on Conjugate Functions.
187.] THEOREM IV. If  and
and  , and also
, and also  and
and  are conjugate functions of
are conjugate functions of  and
and  , then, if
, then, if
 and
and 
 and
and  will be conjugate functions of
will be conjugate functions of  and
and  .
.
For

THEOREM V. If  be a solution of the equation
be a solution of the equation
 ,
,
and if
 , and
, and  ,
,
 and
and  will be conjugate functions of
will be conjugate functions of  and
and  .
.
For  and
and  are conjugate functions of
are conjugate functions of  and
and  , and these are conjugate functions of
, and these are conjugate functions of  and
and  .
.
188.] As an example of the general method of transformation let us take the case of inversion in two dimensions.
If  is a fixed point in a plane, and
is a fixed point in a plane, and  a fixed direction, and if
a fixed direction, and if  , and
, and  , and if
, and if  are the rectangular coordinates of
are the rectangular coordinates of  with respect to
with respect to  ,
,

|
(5)
|
 and
and  are conjugate functions of
are conjugate functions of  and
and  .
.
If  and
and  ,
,  and
and  will be conjugate functions of
will be conjugate functions of  and
and  . In the case in which
. In the case in which  we have
we have
 , ,
|
|
which is the case of ordinary inversion combined with turning the figure 180° round  .
.
Inversion in Two Dimensions.
In this case if  and
and  represent the distances of corresponding
represent the distances of corresponding