is still further diminished. In general one half of the heat received from the sun by the illuminated terrestrial hemisphere is absorbed by the clearest atmosphere, leaving the other half to reach the surface of the ground, provided there be no intercepting clouds. The thermal conditions actually observed at the immediate surface of the globe during hazy and cloudy weather are therefore of minor importance in the mechanism of the whole atmosphere, as compared with the influence of the heat retained within its mass.
The transmission of solar radiation through the earth’s atmosphere is the fundamental problem of meteorology, and has been the subject of many studies, beginning with J. H. Lambert and P. Bouguer. The pyrheliometer of C. S. M. Pouillet gave us our first idea of the thermal equivalent of solar radiation outside of our atmosphere or the so-called “solar constant,” the value of which has been variously placed at from 2 to 4 calories per sq. cm. per minute. At present the weight of the argument is in favour of 2·1, with a fair presumption that both the intensity and the quality of the solar radiation as it strikes the upper layers of our atmosphere are slightly variable. It is also likely that this “constant” does not represent the sun proper, but the remaining energy after the sunbeam has sifted through masses of matter between the sun and our upper atmosphere, so that it may thus come to have appreciable variations:
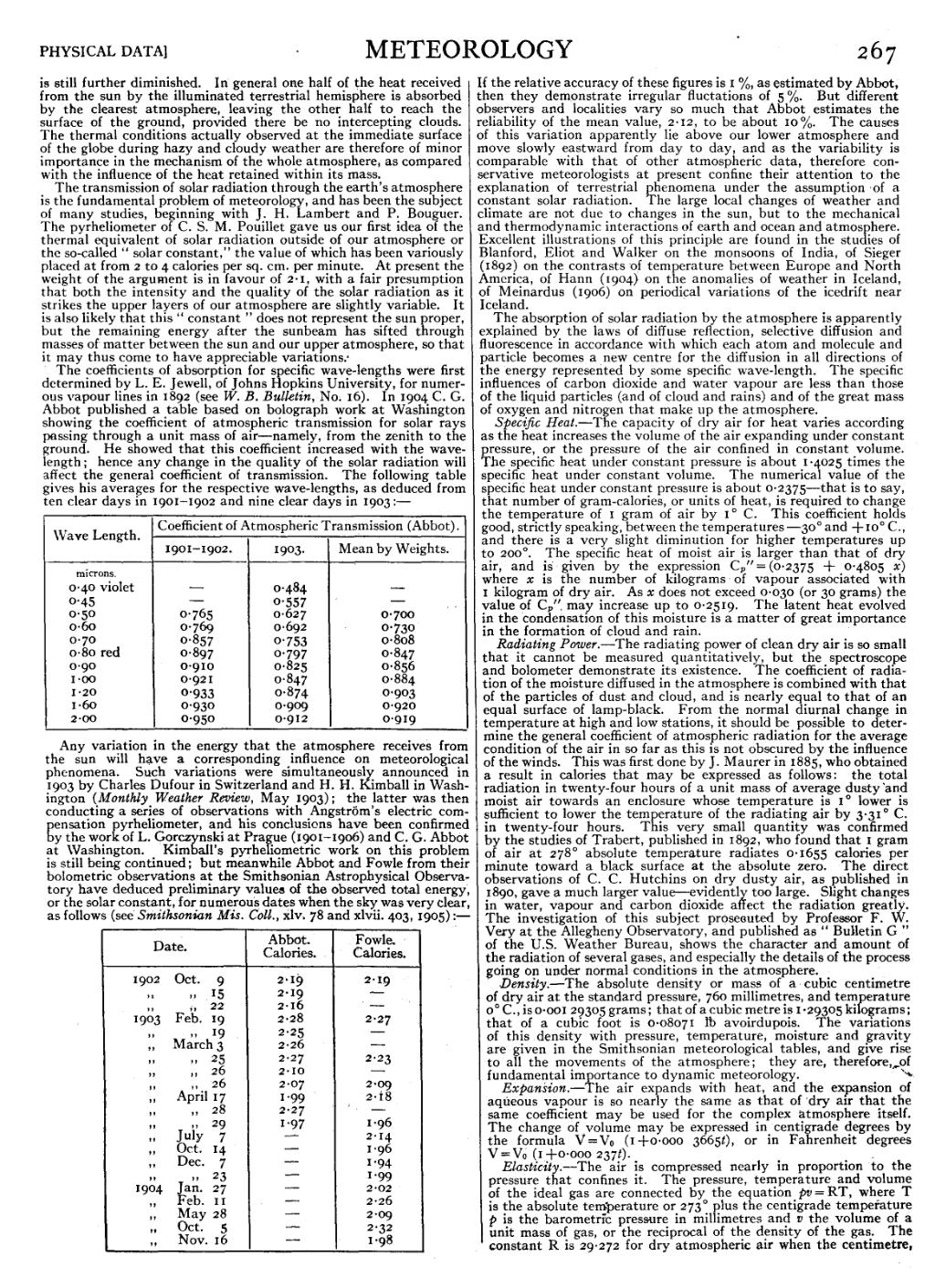
The coefficients of absorption for specific wave-lengths were first determined by L. E. Jewell, of Johns Hopkins University, for numerous vapour lines in 1892 (see W. B. Bulletin, No. 16). In 1904 C. G. Abbot published a table based on bolograph work at Washington showing the coefficient of atmospheric transmission for solar rays passing through a unit mass of air-namely, from the zenith to the ground. He showed that this coefficient increased with the wavelength; hence any change in the quality of the solar radiation will affect the general coefficient of transmission. The following table gives his averages for the respective wave-lengths, as deduced from ten clear days in 1901–1902 and nine clear days in 1903:—
| Wave Length. | Coefficient of Atmospheric Transmission (Abbot). | ||
| 1901–1902. | 1903. | Mean by Weights. | |
| microns. | |||
| 0·40 violet | — | 0·484 | — |
| 0·45 | — | 0·557 | — |
| 0·50 | 0·765 | 0·627 | 0·700 |
| 0·60 | 0·769 | 0·692 | 0·730 |
| 0·70 | 0·857 | 0·753 | 0·808 |
| 0·80 red | 0·897 | 0·797 | 0·847 |
| 0·90 | 0·910 | 0·825 | 0·856 |
| 1·00 | 0·921 | 0·847 | 0·884 |
| 1·20 | 0·933 | 0·874 | 0·903 |
| 1·60 | 0·930 | 0·909 | 0·920 |
| 2·00 | 0·950 | 0·912 | 0·919 |
Any variation in the energy that the atmosphere receives from the sun will have a corresponding influence on meteorological phenomena. Such variations were simultaneously announced in 1903 by Charles Dufour in Switzerland and H. H. Kimball in Washington (Monthly Weather Review, May 1903); the latter was then conducting a series of observations with Angström’s electric compensation pyrheliometer, and his conclusions have been confirmed by the work of L. Gorczynski at Prague (1901–1906) and C. G. Abbot at Washington. Kimball’s pyrheliometric work on this problem is still being continued; but meanwhile Abbot and Fowle from their bolometric observations at the Smithsonian Astrophysical Observatory have deduced preliminary Values of the observed total energy, or the solar constant, for numerous dates when the sky was very clear, as follows (see Smithsonian Mis. Coll., xlv. 78 and xlvii. 403, 1905):—
| Date. | Abbot. Calories. |
Fowle. Calories. |
| 1902 Oct. 9 | 2·19 | 2·19 |
| „ „ 15 | 2·19 | — |
| „ „ 22 | 2·16 | — |
| 1903 Feb. 19 | 2·28 | 2·28 |
| „ „ 19 | 2·25 | — |
| „March 3 | 2·26 | — |
| „ „ 25 | 2·27 | 2·23 |
| „ „ 26 | 2·10 | — |
| „ „ 26 | 2·07 | 2·09 |
| „ April 17 | 1·99 | 2·18 |
| „ „ 28 | 2·27 | — |
| „ „ 29 | 1·97 | — |
| „ July 7 | — | 2·14 |
| „ Oct. 14 | — | 1·96 |
| „ Dec. 7 | — | 1·94 |
| „ „ 23 | — | 1·99 |
| 1904 Jan. 27 | — | 2·02 |
| „ Feb. 11 | — | 2·26 |
| „ May 28 | — | 2·09 |
| „ Oct. 5 | — | 2·32 |
| „ Nov. 16 | — | 1·98 |
If the relative accuracy of these figures is 1%, as estimated by Abbot, then they demonstrate irregular fluctuations of 5%. But different observers and localities vary so much that Abbot estimates the reliability of the mean value, 2·12, to be about 10%. The causes of this variation apparently lie above our lower atmosphere and move slowly eastward from day to day, and as the variability is comparable with that of other atmospheric data, therefore conservative meteorologists at present confine their attention to the explanation of terrestrial phenomena under the assumption of a constant solar radiation. The large local changes of weather and climate are not due to changes in the sun, but to the mechanical and thermodynamic interactions of earth and ocean and atmosphere. Excellent illustrations of this principle are found in the studies of Blanford, Eliot and Walker on the monsoons of India, of Sieger (1892) on the contrasts of temperature between Europe and North America, of Hann (1904) on the anomalies of weather in Iceland, of Meinardus (1906) on periodical variations of the icedrift near Iceland.
The absorption of solar radiation by the atmosphere is apparently explained by the laws of diffuse reflection, selective diffusion and fluorescence in accordance with which each atom and molecule and particle becomes a new centre for the diffusion in all directions of the energy represented by some specific wave-length. The specific influences of carbon dioxide and water vapour are less than those of the liquid particles (and of cloud and rains) and of the great mass of oxygen and nitrogen that make up the atmosphere.
Specific Heat.–The capacity of dry air for heat varies according as the heat increases the volume of the air expanding under constant pressure, or the pressure of the air confined in constant volume. The specific heat under constant pressure is about 1·4025 times the specific heat under constant volume. The numerical value of the specific heat under constant pressure is about 0·2375—that is to say, that number of gram-calories, or units of heat, is required to change the temperature of 1 gram of air by 1° C. This coefficient holds good, strictly speaking, between the temperatures −30° and +10° C., and there is a very slight diminution for higher temperatures up to 200°. The specific heat of moist air is larger than that of dry air, and is given by the expression Cp″=(0·2375 + 0·4805 x) where x is the number of kilograms of vapour associated with 1 kilogram of dry air. As x does not exceed 0·030 (or 30 grams) the value of Cp″ may increase up to 0·2519. The latent heat evolved in the 'condensation of this moisture is a matter of great importance in the formation of cloud and rain.
Radiating Power.—The radiating power of clean dry air is so small that it cannot be measured quantitatively, but the spectroscope and bolometer demonstrate its existence. The coefficient of radiation of the moisture diffused in the atmosphere is combined with that of the particles of dust and cloud, and is nearly equal to that of an equal surface of lamp-black. From the normal diurnal change in temperature at high and low stations, it should be possible to determine the general coefficient of atmospheric radiation for the average condition of the air in so far as this is not obscured by the influence of the winds. This was first done by J. Maurer in 1885, who obtained a result in calories that may be expressed as follows: the total radiation in twenty-four hours of a unit mass of average dusty and moist air towards an enclosure whose temperature is 1° lower is sufficient to lower the temperature of the radiating air by 3·31° C. in twenty-four hours. This very small quantity was confirmed by the studies of Trabert, published in 1892, who found that 1 gram of air at 278° absolute temperature radiates 0·1655 calories per minute toward a black surface at the absolute zero. The direct observations of C. C. Hutchins on dry dusty air, as published in 1890, gave a much larger value—evidently too large. Slight changes in Water, vapour and carbon dioxide affect the radiation greatly. The investigation of this subject prosecuted by Professor F. W. Very at the Allegheny Observatory, and published as “Bulletin G” of the U.S. Weather Bureau, shows the character and amount of the radiation of several gases, and especially the details of the process going on under normal conditions in the atmosphere.
Density.—The absolute density or mass of a cubic centimetre of dry air at the standard pressure, 760 millimetres, and temperature 0° C., is 0·001 29305 grams; that of a cubic metre is 1·29305 kilograms; that of a cubic foot is 0·08071 ℔ avoirdupois. The variations of this density with pressure, temperature, moisture and gravity are given in the Smithsonian meteorological tables, and give rise to all the movements of the atmosphere; they are, therefore, of fundamental importance to dynamic meteorology.
Expansion.—The air expands with heat, and the expansion of aqueous vapour is so nearly the same as that of dry air that the same coefficient may be used for the complex atmosphere itself. The change of volume may be expressed in centigrade degrees by the formula V=V0 (1+0·000 3665t), or in Fahrenheit degrees V=V0 (1 +0·000 237t).
Elasticity.—The air is compressed nearly in proportion to the pressure that confines it. The pressure, temperature and volume of the ideal gas are connected by the equation pv=RT, where T is the absolute temperature or 273° plus the centigrade temperature p is the barometric pressure in millimetres and v the volume of a unit mass of gas, or the reciprocal of the density of the gas. The constant R is 29·272 for dry atmospheric air when the centimetre,