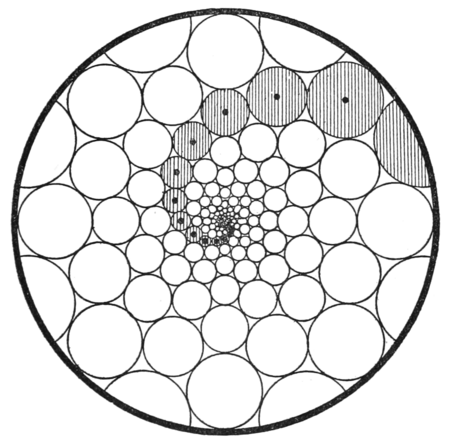
easily be proved that the best distribution of the elements is obtained when the lines appearing in the configuration of the flower (Fig. 5) are logarithmic spirals.[1]
In a similar manner the location of the leaves along the stem of a plant is determined by remarkable numerical relations.[2] The fraction n/m expressing the parts of the circumference by which consecutive leaves are separated are constant for each species and are the successive approximations of the continued fraction
| 1 | ||||||
| 1 | + | 1 | ||||
| 1 | + | 1 | ||||
| 1 | + | ..., | ||||
i. e., 1, 12, 23, 35, 58, 812, …, which, on the other hand, are also the terms of a special Lame's series. Arranging the leaves according to these fractions, nature insures for each species the best distribution of light and masses, and consequently the best growth.
Helical motion in the growth of certain plants around supporting poles may be explained mechanically. The outer parts exposed to the light grow faster than the inner portions, with the resulting tendency to bend the stem around the pole. This, combined with the upward growth, produces the spiral motion. The fact that most climbing plants, with a few exceptions (hops, Polygonum convolvulus, etc.), show left-handed twisting may be circumscribed by calling it "geotropism."