Popular Science Monthly/Volume 13/June 1878/Water-Waves and Sound-Waves
| WATER-WAVES AND SOUND-WAVES.[1] |
By J. NORMAN LOCKYER, F. R. S.
LET us find a piece of tranquil water and drop a stone into it. What happens?—a most beautiful thing, full of the most precious teachings. The place where the stone fell in is immediately surrounded by what we all recognize as a wave of water traveling outward and
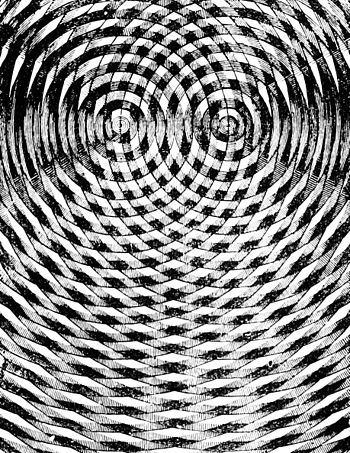
Fig. 1.—Superposition of Two Wave-Systems.
then another is generated, and then another, until at length an exquisite series of concentric waves is seen, all apparently traveling outward —not with uncertain speed, but so regularly that all the waves all round are all parts of circles and of concentric circles.

Fig. 2.—Showing the Formation of Waves by the Circular Motion of Each Particle of Water in a Vertical Plane. Eight positions in each revolution are shown—I. One Particle in Motion.—II. Two Particles in Motion.—III. Three Particles in Motion.—IV. Complete Wave and Motion of its Elements.
Let us drop two stones in at some little distance apart. What happens then? We have two similar systems each working its way outward, to all appearance independently of the other. We get what is represented in Fig. 1.

Fig. 3.—Graphical Method of observing the Mode of Vibration of a Tuning-Fork.
Now, these appearances are as if there were an actual outpouring of water from the cavity made by the stone; but if we strew small pieces of paper or other light material on the water-surface before we drop the stone, we find that it is not the water which moves outward, but only the state of things—the wave. Each particle of water moves in a circular or elliptic path in a vertical plane lying along the direction of the wave, and so comes again to its original place. Hence it is that only the phase goes on—how it goes on will easily be gathered from Fig. 2.
Let us now pass to a disturbance of another kind, from two dimensions to three, from the surface of water to air.
We hear the report of a gun or the screech of a railway-whistle, or any other noise which strikes the ear. How comes it that the ear is struck? Certainly no one will imagine that the sound comes from the cannon or from the railway-whistle like a mighty rush of air. If it came like a wind we should feel it as a wind, but as a matter of fact

Fig. 4.—Shells of Compressed and Rarefied Air produced by a Source of Sound.
no rush of this kind is felt. It is clear, therefore, that we do not get a bodily transmission, so to speak, as we get it in the case of the ball thrown from one boy to the other. We have a state of things passing from the sender of the sound to the receiver; the medium through which the sound passes being the air. A sounding body in the middle of a room, for instance, must send out shells of sound as it were, in all directions, because people above, below, and all round it, would hear the sound. Replace the stone by a tuning-fork. To one prong of this fasten a mirror, and on this mirror throw a powerful beam of light. When this tuning-fork is bowed, and a sound is heard, the light thrown by the attached mirror shows the fork to be vibrating, and when the tuning-fork is moved we get an appearance on the screen which reminds us of the rope; or we may use the fork as shown in Fig. 3, and obtain a wavy record on a blackened cylinder.
Experiment shows that we have at one time a sphere of compression—that is to say, the air is packed closely together; and, again, a sphere

Fig. 5.—Propagation of Sound-Waves along a Cylinder.
of rarefaction, when the particles of air are torn farther apart than they are in the other position. The state of things, then, that travels in the case of sound, is a state of compression and rarefaction of the air. Hence, the particle of air travels differently from the particle of water; it moves backward and forward in a straight line in the direction in which the sound is propagated.

Fig. 6.—Sound-Waves. Particles of air, a, b, c, d, e, are in position 1 at rest. The remaining positions show how they are situated at successive instants, when a continuous series of impulses reaches them from the left. In position 2, e. g., only one particle has begun its oscillation; in position 3, only two; while, in position 6, all are in motion.
The preceding figure (Fig. 6) will show bow this backward-and-forward movement results in the compressions and rarefactions to which reference has been made, in consequence of the impulse having been imparted to one molecule after the other. Owing to the pendulum-like motion of the molecules, their relative positions vary at each instant of time.
Prof. Weinhold has given, in his "Experimental Physics," a good method of obtaining on a plane a mental image of what goes on in a so-called sound-wave, and by the courtesy of Messrs. Longmans I am enabled to give here the illustrations which he employs. After all the particles have been put into motion as shown in Fig. 6, if we graphically represent the backward-and-forward oscillation of a particle by such a wavy line as in Fig. 7, we shall, when we put a large number of such waves side by side, introducing the change of phase, have such an arrangement of wavy lines as is represented in Fig. 8.

Fig. 7
Now, the beauty of Weinhold's illustration consists in this: he almost makes each element of each line—each element representing, of course, a particle of air—appear to be actually in motion by treating the above figures in the following way: He cuts a narrow slit, S S, in a piece of stiff paper, either black or of a dark color, as shown in Fig. 9. He then holds this on the dotted line at the bottom of Fig. 7. "The book is now slowly drawn along in the direction of the arrow, the piece of paper being held in the same position. At first the lower extremity of the curved line in A is seen through the slit; but, as the book is drawn along, the portions to the right and those to the left come successively in view; the small white dot, which is the only visible portion of the curved line, appears as a point which moves first to the right and then to the left, and imitates closely the motion of a vibrating particle of air, the rate of motion being, however, much slower. If, now, the slit be placed over the dotted line" (at the bottom of Fig. 8), "and the book drawn along underneath it in the direction of the arrow, a representation is obtained of the motion of a series of particles of air which are acted on by a number of successive equal undulations or waves. Each particle merely moves a little right and left, and always comes back again to its starting-point; but the condensations and rarefactions, represented by the lines being respectively closer together or farther apart, are gradually transmitted through the whole series of air-particles from one end to the other."[2]
In dwelling upon sound-phenomena, we have the advantage of dealing with phenomena about which Science says she does know something: from a consideration of these known facts we shall be able, slowly but surely, to grasp some of the much less familiar phenomena with which spectrum analysis is especially concerned.

Fig. 8.
We all know that some sounds are what is termed high and others low, a difference which in scientific language is expressed by saying that sounds have a difference in pitch. We know that the difference between a sound which is pitched high and a sound which is pitched low is simply this—that the pulses or waves, as we may call them for simplicity's sake, which go from the sender-forth of the sound (which may be a cannon, a piano, or anything else) to the receiver, which is generally the human ear, are of different lengths. What in physics is called a sound-wave is constructed as follows: We have a line A X, which represents the normal condition of the air through which the sound is traveling, and curves which represent to the eye—first, the relative amounts of compression (+) and rarefaction (—) brought about by the sound in the case of each pulse, and secondly the relationship of this to the actual length of the wave, or, what is the same thing, the time taken for the pulse to travel. Thus we may have long waves and short waves independently of the amount of compression or rarefaction, and much or little compression or rarefaction independently of the length of the wave. We know that the difference between a high note and a low note, whether of the voice or of a musical instrument, is, that the high note we can prove to be produced by a succession of short waves—such pulses as have been described—and the low note by a succession of long waves.
Now, the loudness or softness of a note does not alter its pitch, that is, it does not alter the length of its waves or the rate at which they travel. I can send a wave along the rope either violently or gently,

Fig. 9.
but with the same tension of the rope we shall find that the length of the waves is the same, provided the period of vibration is the same. Hence, then, the other idea added to the idea of pitch.
There is another point which is worth noting, although it is not needful to refer to it in any great detail, and that is, that we know that sound travels with a certain velocity, and that this rate is subject to certain small variations owing to different causes.
We not only have to deal with amplitude—that is, the departure of the and—parts of the curve from the line AX—and velocity, but we have this most important and very beautiful fact (for fact it is),

Fig. 10.—Sound-Waves of Different Lengths and Amplitudes.
which some will have observed for themselves: If a person in a room in which there is a piano sings a note, the string of a piano tuned to that particular note will respond; and, if he sing another note, then another string will reply, the first string being silent. And if the experimenter were skilled enough to sing one by one all the notes to which the strings of the piano are tuned, all the strings would be set into vibration one by one, note for note. This fact may be explained in this way: a piano-wire, or similar sonorous body, which is constructed to do a certain thing—in this case to sound a particular note—always sounds that note when it is called upon in a proper way to do it. Now this is the point: The proper way may be either (1) that a particular vibration should fall upon it, or (2) that it should be set to work to generate that vibration in itself. If the piano-wire only gives the same sound when struck either hard or soft, it is because it is manufactured to do one particular kind of work, and it can do no other.
Now we may pass from a piano-wire to a tuning-fork. We find that, by using different quantities or different shapes of metal, these instruments give out different notes. If all be of the same metal, the different quantities of metal will give us a difference in the pitch. This demonstrates that the pitch of a note is independent of any particular quality of the substance set into vibration. Now, although a great many musical instruments can sound the same note, yet the music, the tone, which one gets out of them is very different. That is, the pitch being the same, the quality of the note changes because the wave, or rather the system of waves, which we obtain is different. For instance, if we sound a note upon the violin, or the French horn, or the flute, or the clarionet, anybody who knows anything of music will tell which is in question, so that here we have in addition to wave-length and wave-amplitude another attribute, namely, that which in French is called "timbre," in German "Klangfarbe," and in English, "tone" or "quality."

