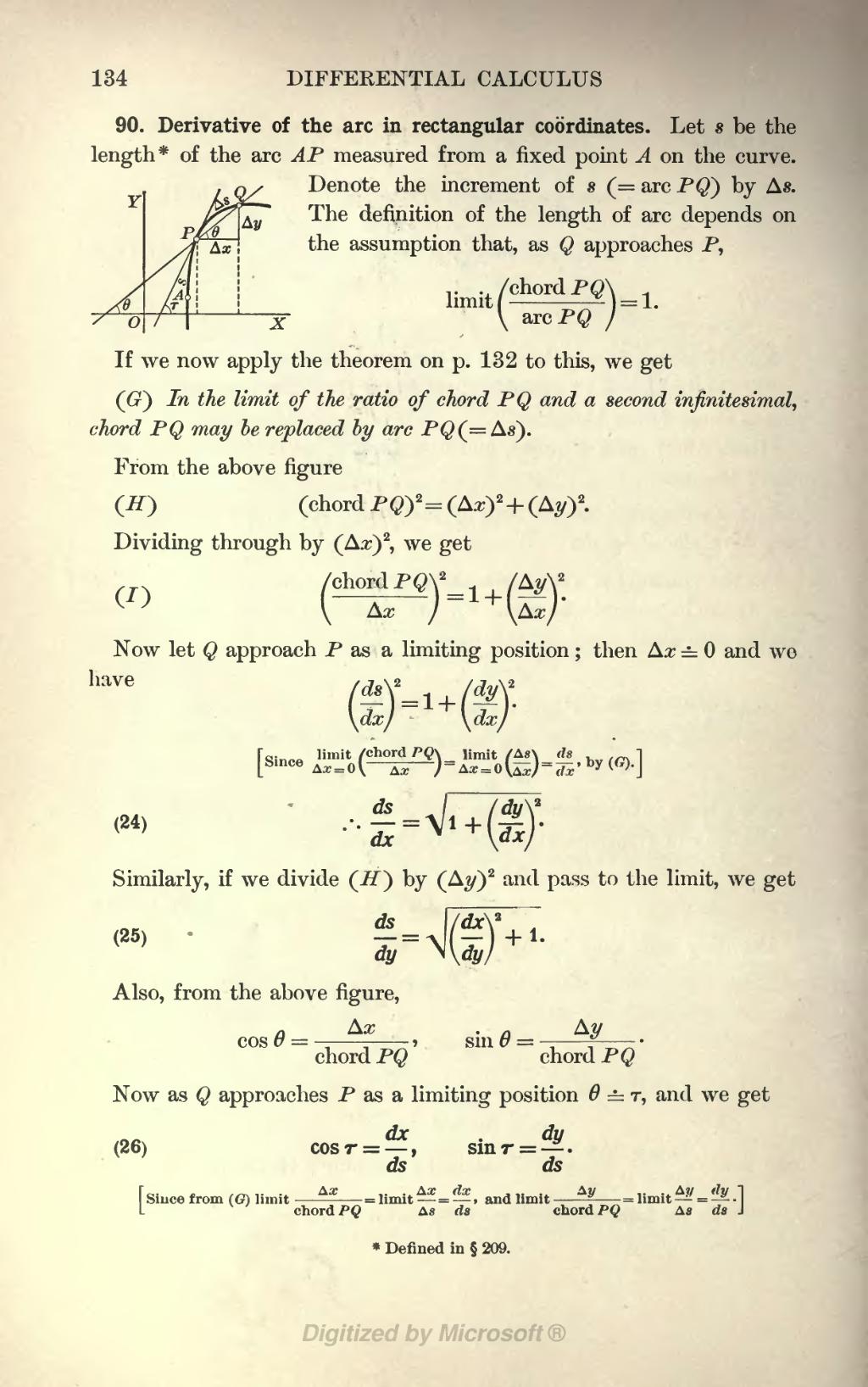
90. Derivative of the arc in rectangular coördinates. Let s be the length[1] of the arc AP measured from a fixed point A on the curve.
 Denote the increment of s (= arc PQ) by
Denote the increment of s (= arc PQ) by  . The definition of the length of arc depends on the assumption that, as Q approaches P,
. The definition of the length of arc depends on the assumption that, as Q approaches P,

If we now apply the theorem in §89 to this, we get
| (G)
|
In the limit of the ratio of chord PQ and a second infinitesimal, chord PQ may be replaced by arc PQ (=  ). ).
|
From the above figure
| (H)
|

|
Dividing through by  , we get
, we get
| (I)
|
 . .
|
Now let Q approach P as a limiting position; then  and we have
and we have
 .
.
[Since  , (G).]
, (G).]
| (24)
|
∴ 
|
Similarly, if we divide (H) by  and pass to the limit, we get
and pass to the limit, we get
| (25)
|

|
Also, from the above figure,


Now as Q approaches P as a limiting position  , and we get
, and we get
| (26)
|
 , , 
|
[Since from (G)  , and
, and  .]
.]
- ↑ Defined in § 209.
 Denote the increment of s (= arc PQ) by . The definition of the length of arc depends on the assumption that, as Q approaches P,
Denote the increment of s (= arc PQ) by . The definition of the length of arc depends on the assumption that, as Q approaches P,